Long Questions (Question 10)

Question 10 (7 marks):
Solution by scale drawing is not accepted.
Diagram shows the locations of town M and town N drawn on a Cartesian plane.


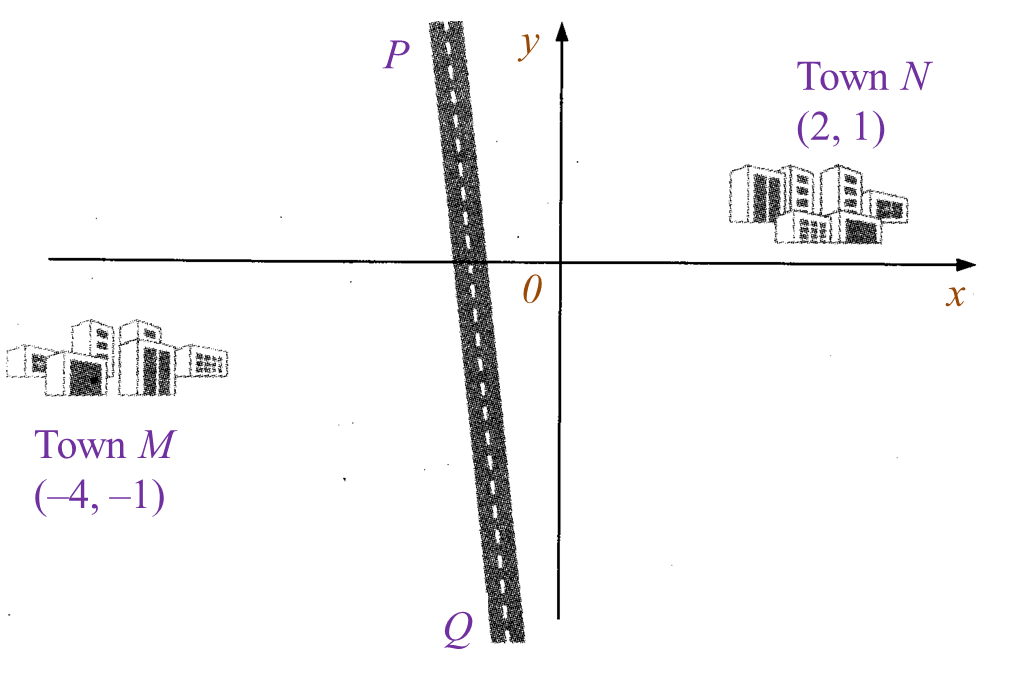
PQ is a straight road such that the distance from town M and town N to any point on the road is always equal.
(a) Find the equation of PQ.

(b) Another straight road, ST with an equation y = 2x + 7 is to be built.
(i) A traffic light is to be installed at the crossroads of the two roads.
Find the coordinates of the traffic light.
(ii) Which of the two roads passes through town L $\left(-\frac{4}{3},1\right)?$

Solution:
(a)
$\begin{array}{l}T\left(x,y\right)\text{ is a point on }PQ.\\ TM=TN\\ \sqrt{\left[x-{\left(-4\right)}^2\right]+{\left[y-\left(-1\right)\right]}^2}=\sqrt{{\left(x-2\right)}^2+{\left(y-1\right)}^2}\\ \sqrt{{\left(x+4\right)}^2+{\left(y+1\right)}^2}=\sqrt{{\left(x-2\right)}^2+{\left(y-1\right)}^2}\\ {\left(x+4\right)}^2+{\left(y+1\right)}^2={\left(x-2\right)}^2+{\left(y-1\right)}^2\\ x^2+8x+16+y^2+2y+1\\ =x^2-4x+4+y^2-2y+1\\ 8x+2y+17+4x+2y-5=0\\ 12x+4y+12=0\\ 3x+y+3=0\\ \\ \text{Equation of }PQ:3x+y+3=0\end{array}$


(b)(i)
$\begin{array}{l}y=2x+7\mathrm{............}\left(1\right)\\ 3x+y+3=0\mathrm{............}\left(2\right)\\ \text{Substitute }\left(1\right)\text{ into }\left(2\right):\\ 3x+2x+7+3=0\\ 5x=-10\\ x=-2\\ \\ \text{When }x=-2,\\ From\left(1\right),\\ y=2\left(-2\right)+7=3\\ \text{Coordinates of traffic light}=\left(-2,3\right).\end{array}$


(b)(ii)
$\begin{array}{l}L\left(-\frac{4}{3},1\right):x=-\frac{4}{3},y=1\\ \text{The equation of }ST:y=2x+7\\ \text{Left hand side: }y=1\\ \text{Right hand side: }2\left(-\frac{4}{3}\right)+7=4\frac{1}{3}\\ \text{Thus, the road }y=2x+7\text{ does not }\\ \text{pass through }L\text{.}\\ \\ \text{The equation of }PQ:3x+y+3=0\\ \text{Left hand side: }\\ 3x+y+3=3\left(-\frac{4}{3}\right)+1+3\\ =-4+4=0\\ \text{Right hand side}=0\\ \text{Left hand side}=\text{Right hand side}\\ \\ \text{Thus, the road }3x+y+3=0\\ \text{passes through }L\text{.}\end{array}$