Long Questions (Question 11)

Question 11 (10 marks):
A study shows that the credit card balance of the customers is normally distributed as shown in Diagram 6.

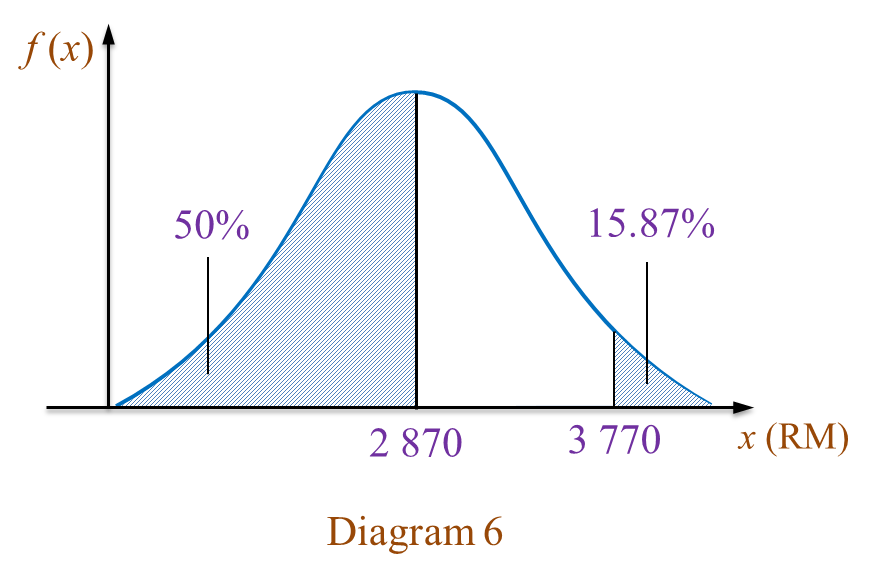(a)(i) Find the standard deviation.
(ii) If 30 customers are chosen at random, find the number of customers who have a credit card balance between RM1800 and RM3000.

(b) It is found that 25% of the customers have a credit card balance less than RM y.
Find the value of y.

Solution:
(a)(i)
$\begin{array}{l}\mu =2870,x=3770\\ P\left(X>3770\right)=15.87\%\\ P\left(Z>\frac{3770-2870}{\sigma }\right)=0.1587\\ P\left(Z>1.0\right)=0.1587\\ \frac{3770-2870}{\sigma }=1.0\\ \sigma =900\end{array}$


(a)(ii)
$\begin{array}{l}P\left(1800<X<3000\right)\\ =P\left(\frac{1800-2870}{900}<Z<\frac{3000-2870}{900}\right)\\ =P\left(-1.189<Z<0.144\right)\\ =1-P\left(Z\le -1.189\right)-P\left(Z\ge 0.144\right)\\ =1-0.1172-0.4427\\ =0.4401\\ \\ \text{Number of customers}=0.4401\times 30\\ =14\end{array}$


(b)
$\begin{array}{l}\mu =2870,x=y\\ P\left(x<y\right)=25\%\\ P\left(Z<\frac{y-2870}{900}\right)=0.25\\ \frac{y-2870}{900}=-0.674\\ y=2263.40\end{array}$