Home › SPM Add Maths › Probability Distribution › Short Questions (Question 3 & 4)

Short Questions (Question 3 & 4)

Posted on by

Question 3 (4 marks):
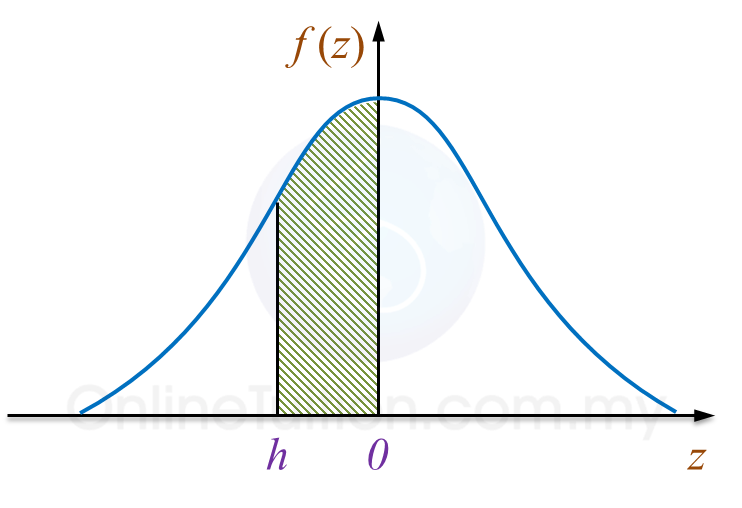
Diagram shows a standard normal distribution graph.

Diagram

The probability represented by the area of the shaded region is 0.2881.
(a) Find the value of h.
(b) X is a continuous random variable which is normally distributed with a mean, μ and a variance of 16.
Find the value of μ if the z-score of X = 58.8 is h.


Solution:
(a)
P(X < h) = 0.5 – 0.2881
P(X < h) = 0.2119
P(X < –0.8) = 0.2119
h = –0.8

(b)

X=58.8 X−μ σ = 58.8−μ σ    Z= 58.8−μ 4    h= 58.8−μ 4 −0.8= 58.8−μ 4 −3.2=58.8−μ μ=58.8+3.2 μ=62



Question 4 (4 marks):
A voluntary body organizes a first aid course 4 times per month, every Saturday from March until September.
[Assume there are four Saturdays in every month]

Salmah intends to join the course but she might need to spare a Saturday per month to accompany her mother to the hospital. The probability that Salmah will attend the course each Saturday is 0.8. Salmah will be given a certificate of monthly attendance if she can attend the course at least 3 times a month.

(a) Find the probability that Salmah will be given the certificate of monthly attendance.

(b) Salmah will qualify to sit for the first aid test if she obtains more than 5 certificates of monthly attendance.
Find the probability that Salmah qualifies to take the first aid test.


Solution:
(a)

P( X=r )= C n r p r q n−r p=0.8, q=0.2, n=4, r=3, 4 P( X≥3 ) =P( X=3 )+P( X=4 ) = C 4 3 ( 0.8 ) 3 ( 0.2 ) 1 + C 4 4 ( 0.8 ) 4 ( 0.2 ) 0 =0.4096+0.4096 =0.8192

(b)

P( X=r )= C n r p r q n−r p=0.8192, q=0.1808, n=7, r=6, 7 P( X>5 ) =P( X=6 )+P( X=7 ) = C 7 6 ( 0.8192 ) 6 ( 0.1808 ) 1 + C 7 7 ( 0.8192 ) 7 ( 0.1808 ) 0 =0.3825+0.2476 =0.6301