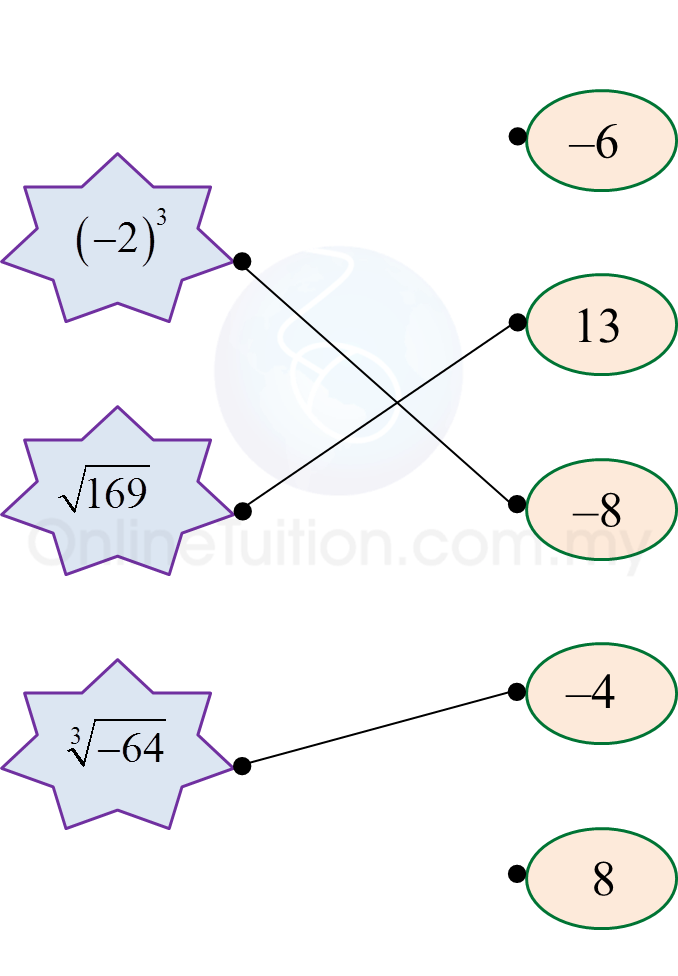
Question 6:
Simplify −4(5x−3)+7x−1.
Solution:
−4(5x−3)+7x−1=−20x+12+7x−1=−13x+11
Simplify −4(5x−3)+7x−1.
Solution:
−4(5x−3)+7x−1=−20x+12+7x−1=−13x+11
Question 7:
(3x−2y)−(x+4y)
Solution:
(3x−2y)−(x+4y)=3x−2y−x−4y=2x−6y
(3x−2y)−(x+4y)
Solution:
(3x−2y)−(x+4y)=3x−2y−x−4y=2x−6y
Question 8:
Simplify −3(2a−4b)−13(6a−15b)
Solution:
−3(2a−4b)−13(6a−15b)=−6a+12b−2a+5b=−8a+17b
Simplify −3(2a−4b)−13(6a−15b)
Solution:
−3(2a−4b)−13(6a−15b)=−6a+12b−2a+5b=−8a+17b
Question 9:
13(−2x+6y−9z)−16(−4x−18y+24z)
Solution:
13(−2x+6y−9z)−16(−4x−18y+24z)=−23x+2y−3z+23x+3y−4z=5y−7z
13(−2x+6y−9z)−16(−4x−18y+24z)
Solution:
13(−2x+6y−9z)−16(−4x−18y+24z)=−23x+2y−3z+23x+3y−4z=5y−7z
Question 10:
12(a+6bc)−15(3+2bc−2a)
Solution:
12(a+6bc)−15(3+2bc−2a)=12a+3bc−35−25bc+25a=5a+4a10+15bc−2bc5−35=9a10+13bc5−35
12(a+6bc)−15(3+2bc−2a)
Solution:
12(a+6bc)−15(3+2bc−2a)=12a+3bc−35−25bc+25a=5a+4a10+15bc−2bc5−35=9a10+13bc5−35