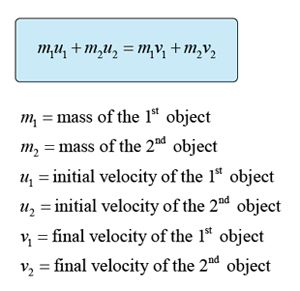
Principle of Conservation of Momentum
The principle of conservation of momentum states that in a system make out of objects that react (collide or explode), the total momentum is constant if no external force is acted upon the system.Sum of Momentum Before Reaction
= Sum of Momentum After Reaction
Formula
Example - Both Object are in the Same Direction before Collision
A Car A of mass 600 kg moving at 40 ms-1 collides with a car B of mass 800 kg moving at 20 ms-1 in the same direction. If car B moves forwards at 30 ms-1 by the impact, what is the velocity, v, of the car A immediately after the crash?
Answer:
m1 = 600kg
m2 = 800kg
u1 = 40 ms-1
u2 = 20 ms-1
v1 = ?
v2 = 30 ms-1
According to the principle of conservation of momentum,
m1u1 + m2u2 = m1v1 + m2v2
(600)(40) + (800)(20) = (600)v1 + (800)(30)
40000 = 600v1 + 24000
600v1 = 16000
v1 = 26.67 ms-1
A Car A of mass 600 kg moving at 40 ms-1 collides with a car B of mass 800 kg moving at 20 ms-1 in the same direction. If car B moves forwards at 30 ms-1 by the impact, what is the velocity, v, of the car A immediately after the crash?
Answer:
m1 = 600kg
m2 = 800kg
u1 = 40 ms-1
u2 = 20 ms-1
v1 = ?
v2 = 30 ms-1
According to the principle of conservation of momentum,
m1u1 + m2u2 = m1v1 + m2v2
(600)(40) + (800)(20) = (600)v1 + (800)(30)
40000 = 600v1 + 24000
600v1 = 16000
v1 = 26.67 ms-1
Example - Both Object are in opposite direction Before Collision
A 0.50kg ball traveling at 6.0 ms-1 collides head-on with a 1.0 kg ball moving in the opposite direction at a speed of 12.0 ms-1. The 0.50kg ball moves backward at 14.0 ms-1 after the collision. Find the velocity of the second ball after collision.
Answer:
m1 = 0.5 kg
m2 = 1.0 kg
u1 = 6.0 ms-1
u2 = -12.0 ms-1
v1 = -14.0 ms-1
v2 = ?
(IMPORTANT: velocity is negative when the object move in opposite direction)
According to the principle of conservation of momentum,
m1u1 + m2u2 = m1v1 + m2v2
(0.5)(6) + (1.0)(-12) = (0.5)(-14) + (1.0)v2
-9 = - 7 + 1v2
v2 = -2 ms-1
A 0.50kg ball traveling at 6.0 ms-1 collides head-on with a 1.0 kg ball moving in the opposite direction at a speed of 12.0 ms-1. The 0.50kg ball moves backward at 14.0 ms-1 after the collision. Find the velocity of the second ball after collision.
Answer:
m1 = 0.5 kg
m2 = 1.0 kg
u1 = 6.0 ms-1
u2 = -12.0 ms-1
v1 = -14.0 ms-1
v2 = ?
(IMPORTANT: velocity is negative when the object move in opposite direction)
According to the principle of conservation of momentum,
m1u1 + m2u2 = m1v1 + m2v2
(0.5)(6) + (1.0)(-12) = (0.5)(-14) + (1.0)v2
-9 = - 7 + 1v2
v2 = -2 ms-1
Elastic and Inelastic Collision
Elastic Collision
Elastic collision is the collision where the kinetic energy is conserved after the collision.Total Kinetic Energy before Collision
= Total Kinetic Energy after Collision
Additional notes:
- In an elastic collision, the 2 objects separated right after the collision, and
- the momentum is conserved after the collision.
- Total energy is conserved after the collision.
Inelastic Collision
Inelastic collision is the collision where the kinetic energy is not conserved after the collision.Additional notes:
- In a perfectly elastic collision, the 2 objects attach together after the collision, and
- the momentum is also conserved after the collision.
- Total energy is conserved after the collision.
Example - Perfectly Inelastic Collision:
A lorry of mass 8000kg is moving with a velocity of 30 ms-1. The lorry is then accidentally collides with a car of mass 1500kg moving in the same direction with a velocity of 20 ms-1. After the collision, both the vehicles attach together and move with a speed of velocity v. Find the value of v.
Answer:
(IMPORTANT: When 2 object attach together, they move with same speed.)
m1 = 8000kg
m2 = 1500kg
u1 = 30 ms-1
u2 = 20 ms-1
v1 = v
v2 = v
According to the principle of conservation of momentum,
m1u1 + m2u2 = m1v1 + m2v2
(8,000)(30) + (1,500)(20) = (8,000)v+ (1,500)v
270,000 = 9500v
v = 28.42 ms-1
A lorry of mass 8000kg is moving with a velocity of 30 ms-1. The lorry is then accidentally collides with a car of mass 1500kg moving in the same direction with a velocity of 20 ms-1. After the collision, both the vehicles attach together and move with a speed of velocity v. Find the value of v.
Answer:
(IMPORTANT: When 2 object attach together, they move with same speed.)
m1 = 8000kg
m2 = 1500kg
u1 = 30 ms-1
u2 = 20 ms-1
v1 = v
v2 = v
According to the principle of conservation of momentum,
m1u1 + m2u2 = m1v1 + m2v2
(8,000)(30) + (1,500)(20) = (8,000)v+ (1,500)v
270,000 = 9500v
v = 28.42 ms-1