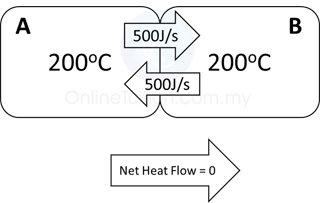
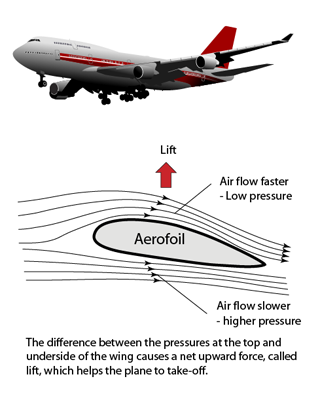
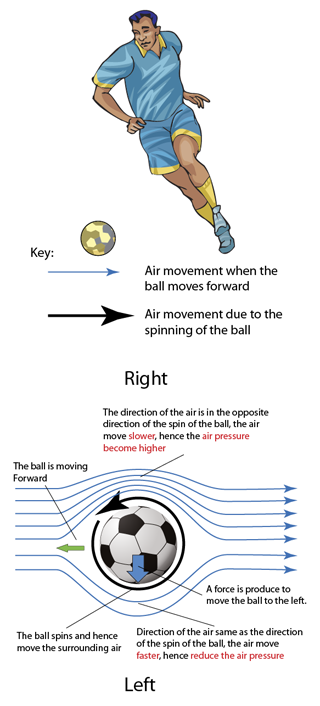
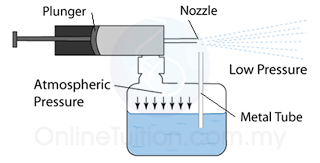
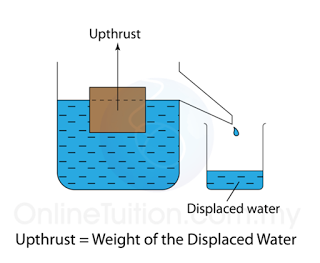
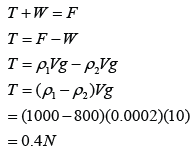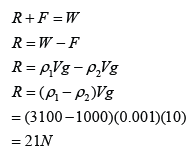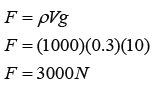Calibrating a Thermometer
- To calibrate a thermometer means to put the correct mark of reading at the correct place so that other temperature can be deduced from these marks.
- To do this, two extreme points are chosen to mark its scale and these points must be able to be reproduced accurately.
- Usually, we take the steam point of pure water as 100°C and the ice point of water as 0°C.

To calibrate a thermometer, the ice point of water is usually taken to be 0°C 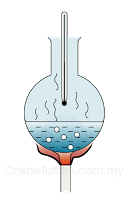
To calibrate a thermometer, the steam point is taken to be 100°C
- After determining the position of the ice point and steam point, the temperature of an object can be determined by using the formula:
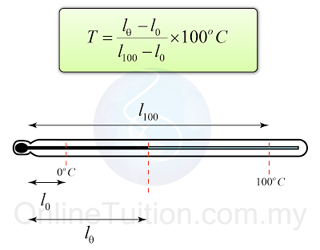
Example 1:
The length of the mercury column in a non-calibrated mercury thermometer is 2cm when its bulb is immerse in melting ice and 20cm when the bulb is in steam above boiling water. What would the temperature be is the length of the mercury column is 11cm?
Answer:
l0°C = 2cm
l100°C = 20cm
lθ = 11cm
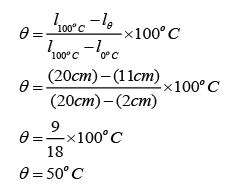
The length of the alcohol column in a thermometer is 2.5cm and 17.5cm when the thermometer is dipped into a melting ice and a boiling water respectively. Find the distance between every 10°C of the scale on the thermometer.
Answer:
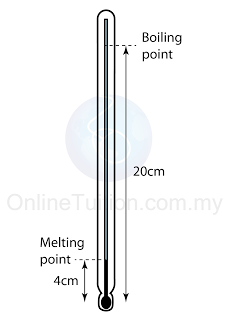
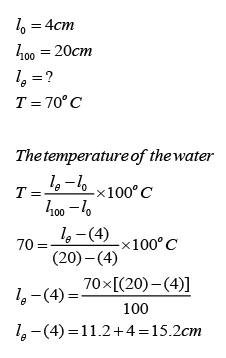
Figure above shows the length of the mercury thread of a thermometer at melting point and boiling point of water. What is the length of the mercury thread when the thermometer is dipped into a hot liquid of temperature 70°C?
Answer:
Absolute Zero and the Kelvin Temperature Scale
- Absolute temperature is the temperature measured in Kelvin scale, which it is a temperature reading made relative to absolute zero.
- We can convert a temperature in °C to absolute temperature by adding 273 to the temperature.
For example:
25°C = 273 + 25 = 298 K
100°C = 273 + 100 = 373 K - Absolute zero is the temperature where thermal energy is at minimum. It is 0 on the Kelvin scale and -273 on the Celsius scale.