5.7 SPM Practice (Long Questions 3)

Posted on April 23, 2020 by user

Question 5:

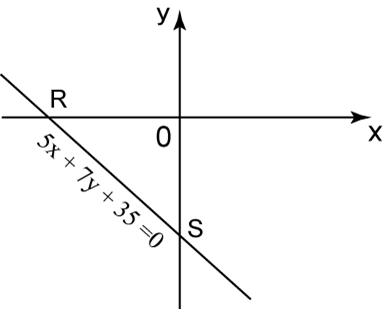
In the diagram above, a straight line 5x + 7y + 35 = 0 intersects with the x-axis and y-axis at R and S respectively. Determine

(a) the gradient of the straight line RS.

(b) the x-intercept of the straight line RS.

(c) the distance of RS.

Solution:
(a)

$\begin{array}{l} 5 x + 7 y + 35 = 0 \\ 7 y = - 5 x - 35 \\ y = - \frac{5}{7} x - 5 \\ ∴ The gradient of the straight line R S = - \frac{5}{7} . \end{array}$

(b)

$\begin{array}{l} At x-intercept, y = 0 \\ 0 = - \frac{5}{7} x - 5 \\ \frac{5}{7} x = - 5 \\ x = - 7 \\ ∴ x-intercept of the straight line R S = - 7. \end{array}$

(c)

$\begin{array}{l} Point R = (- 7, 0) and point S = (0, - 5) \\ Distance of R S = \sqrt{{(- 7 - 0)}^{2} + {(0 - (- 5))}^{2}} \\ Distance of R S = \sqrt{49 + 25} \\ Distance of R S = \sqrt{74} units \end{array}$

Question 6:

In the diagram above, O is the origin of the Cartesian plane, AOB is a straight line and OA = AC. Find

(a) the coordinates of C.

(b) the value of h.

(c) the equation of BC.

Solution:
(a)

x-coordinate of C = –3 × 2 = –6

Therefore coordinates of C = (–6, 0).

(b)

$\begin{array}{l} Gradient of A O = Gradient of O B \\ \frac{0 - (- 4)}{0 - (- 3)} = \frac{h - 0}{6 - 0} \\ \frac{4}{3} = \frac{h}{6} \\ h = 8 \end{array}$

(c)

$\begin{array}{l} Gradient of B C = \frac{8 - 0}{6 - (- 6)} = \frac{8}{12} = \frac{2}{3} \\ At point C (- 6, 0), \\ 0 = \frac{2}{3} (- 6) + c \\ c = 4 \\ The equation of B C is, \\ y = \frac{2}{3} x + 4 \end{array}$