Home › PT3 Maths › Archive for Linear Equations II

Linear Equations II

11.2.2 Linear Equations II, PT3 Focus Practice

Posted on by

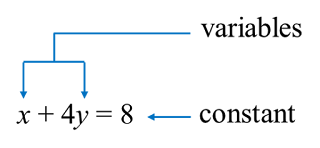
Question 6:
Diagram below shows 5 boxes of orange juice and 2 bottles of milk. Both orange juice and milk are mixed to produce 18 litres drink.


(a) Based on the above situation, write a linear equation.
(b) If 9 litres of drinks is produced by using 2 boxes of orange juice and 2 bottles of milk, find the volume, in litre, of orange juice in each box.

Solution:
Let j be the number of boxes of orange juice and m be the number of bottles of milk.
(a)
5j + 2m = 18

(b)
2j+2m=9  ............. ( 1 ) 5j+2m=18 ............. ( 2 ) ( 2 )−( 1 ): 3j=9 j= 9 3 =3 3 litres of orange juice in each box.


11.2.1 Linear Equations II, PT3 Focus Practice

Posted on by

11.2.1 Linear Equations II, PT3 Focus Practice

Question 1:
It is given that 2x = 6 and 3x + y = 10.
Calculate the value of y.

Solution:
2x = 6
x = 3
Substitute x = 3 into 3x + y = 10
3 (3) + y = 10
y = 10 – 9
y = 1


Question 2:
It is given that y = –1 and x – 3y = –10.
Calculate the value of x.

Solution:
Substitute y = –1 into x – 3y = –10
x – 3 (–1) = –10
x + 3 = –10
x = –10 – 3
x = –13


Question 3:
It is given that 7x – 2y = 19 and x + y = 13.
Calculate the value of y.

Solution:
Using Substitution method
7x – 2y = 19 -------- (1)
x + y = 13 ------- (2)
From equation (2),
x = 13 – y ------- (3)
Substitute equation (3) into equation (1),
7x – 2y = 19
7 (13 – y) – 2y = 19
91 – 7y – 2y = 19
– 9y = 19 – 91
– 9y = –72
 y = 8 


Question 4:
It is given that 2x – y = 5 and 3x – 2y = 8.
Calculate the value of x.

Solution:
Using Elimination method
2x – y = 5 -------- (1)
3x – 2y = 8 ------- (2)
(1) × 2:  4x – 2y = 10 -------- (3)
   3x – 2y = 8 ------- (2)
(3) – (2): x – 0 = 2
  x = 2


Question 5:
It is given that x + 2y = 4 and x + 6y = –4.
Calculate the value of x.

Solution:
Using Elimination method
x + 2y = 4 -------- (1)
x + 6y = –4 ------- (2)
(1) × 3:  3x + 6y = 12 -------- (3)
   x + 6y = –4 ------- (2)
(3) – (2): 2x = 12 – (–4)
2x = 16
  x = 8

11.1 Linear Equations II

Posted on by

11.1 Linear Equations II
 
11.1.1 Linear Equations in Two Variables
1.   A linear equation in two variables is an equation which contains only linear terms and involves two variables.
 
 

2.   If the value of one variable in an equation is known, then the value of the other variable can be determined and vice versa.
Example:
Given that 2x + 3y = 6, find the value of
 (a) x when y = 4, (b) y when x = –3

Solution:
(a) Substitute y = 4 into the equation.
 2x + 3y = 6
 2x + 3 (4) = 6
 2x + 12 = 6
 2x = 6 – 12
 2x = –6
x = –3
 
(b)   Substitute x = –3 into the equation.
2x + 3y = 6
2 (–3) + 3y = 6
 –6 + 3y = 6
 3y = 6 + 6
 3y = 12
y = 4
 
 3.   A linear equation in two variables has many possible solutions.


11.1.2  Simultaneous Linear Equations in Two Variables
1.  Two equations are said to be simultaneous linear equations in two variables if
 (a) Both are linear equations in two variables, and
 (b) Both involve the same variables.
Example: 2x + y = 9, x = 2y + 1
 
2.   The solution of two simultaneous linear equations in two variables is any pair of values (x, y) that satisfied both the equations.
3.   Simultaneous linear equations in two variables can be solved by the substitution method or the elimination method.

Example:
Solve the following simultaneous linear equation.
2x + y = 9
3x – y = –4

Solution:
(A)  Substitution method
2x + y = 9 -------- (1) ← label the equations as (1) and (2)
3x – y = –4 ------- (2)
 
From equation (1),
y = 9 – 2x ------- (3) ← expressing y in terms of x.
Substitute equation (3) into equation (2),
3x – (9 – 2x) = –4
3x – 9 + 2x = –4
5x = –4 + 9
5x = 5
x = 1
 
Substitute x = 1 into equation (1),
2 (1) + y = 9
2 + y = 9
  y = 9 – 2
  y = 7
The solution is x = 1, y = 7.


(B) Elimination method
2x + y = 9 -------- (1) ← Both equations have the same coefficient of y.
3x – y = –4 ------- (2)
(1) + (2): 2x + 3x = 9 + (–4) ← y + (–y) = 0
5x = 5
x = 1
 
Substitute x = 1 into equation (1) or (2),
2x + y = 9 -------- (1)
2 (1) + y = 9
 y = 9 – 2
 y = 7  

The solution is x = 1, y = 7.