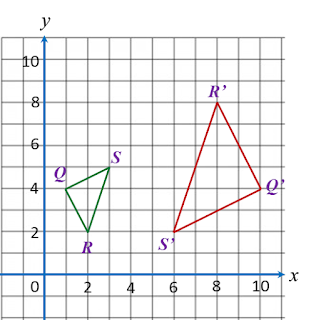
10.2.2 Transformations II, PT3 Focus Practice

Posted on April 21, 2018 by user

Question 6:
Diagram below shows a ‘Wayang Kulit’ performed by Tok Dalang. The height of the screen use is 1.5 m. The shadow on the screen is ⅔ the height of the screen.

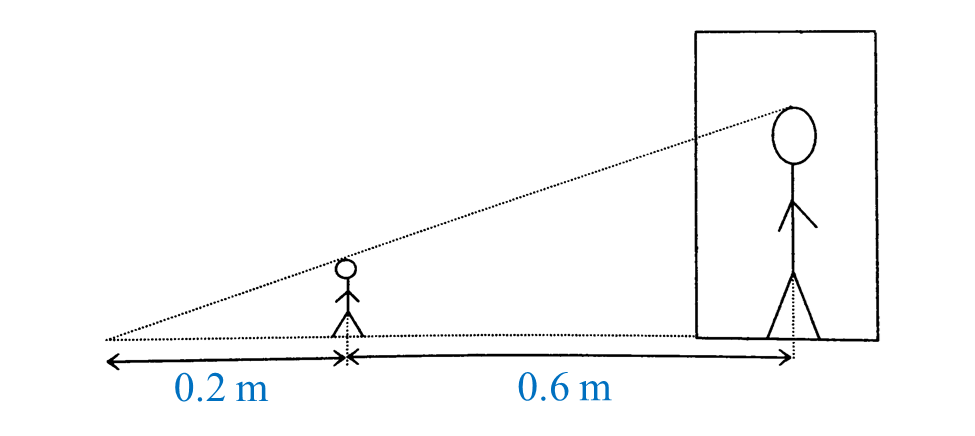What is the height, in cm, of the puppet used by Tok Dalang?

Solution:

\begin{array}{l} Height of shadow \\ = \frac{2}{3} of height of screen \\ = \frac{2}{3} \times 1.5 m \\ = 1.0 m \\ \frac{Height of puppet}{Height of shadow} = \frac{0.2}{0.2 + 0.6} \\ \frac{Height of puppet}{1} = \frac{0.2}{0.8} \\ Height of puppet \\ = 0.25 m \\ = 25 cm \end{array}

Question 7:
Diagram below shows the shadow of a pillar form on the wall from the light of a spotlight.

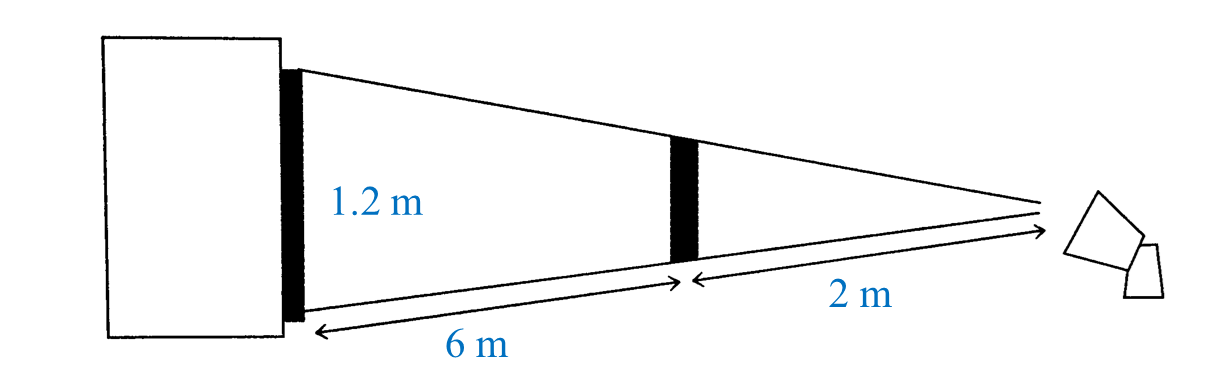(a) State the scale factor of the enlargement.
(b) Find the height of the pillar.

Solution:
(a)

\begin{array}{l} Scale factor = \frac{2 + 6}{2} \\ = \frac{8}{2} \\ = 4 \end{array}

(b)

\begin{array}{l} \frac{Height of pillar}{Height of shadow} = \frac{1}{4} \\ \frac{Height of pillar}{1.2} = \frac{1}{4} \\ Height of pillar = \frac{1}{4} \times 1.2 m \\ = 0.3 m \end{array}

Question 8:
In diagram below, a torch light is used to form a shadow of a candle on the screen.

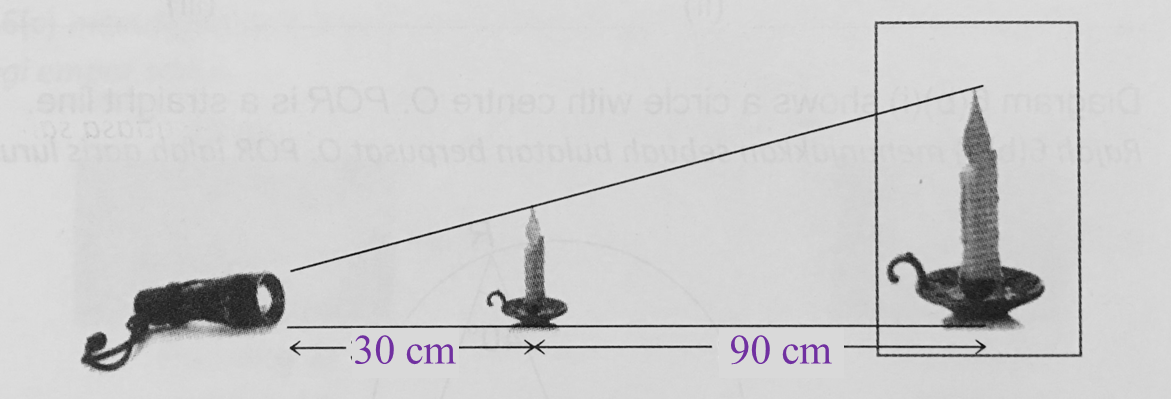
The height of the candle is 15 cm.
If the height of the shadow of the candle is ¾ of the height of the screen, find the height of the screen.

Solution:

\begin{array}{l} \frac{Height of candle}{Height of shadow} = \frac{30}{30 + 90} \\ \frac{15 cm}{Height of shadow} = \frac{1}{4} \\ Height of shadow = 15 cm \times 4 \\ = 60 cm \\ \frac{3}{4} of height of screen = 60 cm \\ Height of screen = \frac{4}{3} \times 60 cm \\ = 80 cm \end{array}

10.2.1 Transformations II, PT3 Focus Practice

Posted on April 19, 2018 by user

10.2.1 Transformations II, PT3 Focus Practice

Question 1:

In the diagram, ∆P’Q’R’ is the image of ∆PQR under an enlargement.

(a) State the scale factor of the enlargement.

(b) If the area of ∆PQR = 7 cm², calculate the area of ∆P’Q’R’.

Solution:

(a)

Scale factor, k = \frac{P' Q'}{P Q} = \frac{6}{3} = 2

(b)

Area of image = k²× Area of object

Area of ∆P’Q’R’ = 2²× 7

= 28 cm²

Question 2:

In diagram below, OP’Q’ is the image of OPQ under an enlargement with centre O.

Given PQ = 4cm, calculate the length, in cm, of P’Q’.

Solution:

\begin{array}{l} \frac{P' Q'}{P Q} = \frac{O P'}{O P} \\ \frac{P' Q'}{4} = \frac{9}{3} \\ P' Q' = \frac{9}{3} \times 4 \\ = 12 cm \end{array}

Question 3:

In diagram below, PQ’R’S’ is the image of PQRS under an enlargement.

Calculate the length, in cm, of SS’.

Solution:

\begin{array}{l} \frac{P S}{P S'} = \frac{P Q}{P Q'} \\ \frac{P S}{36} = \frac{4}{16} \\ P S = \frac{4}{16} \times 36 \\ = 9 cm \\ S S' = 36 - 9 \\ = 27 cm \end{array}

Question 4:

On the Cartesian plane, Q’R’S’ is the image of ∆ QRS under an enlargement of centre T.

State the coordinates of T.

Solution:

Coordinates of T = (4, 4).

Question 5:

In diagram below, quadrilateral AFGH is the image of ABCD under an enlargement.

(a) Find the scale factor of the enlargement.

(b) The area represented by the quadrilateral AFGH is 15cm². Find the area, in cm², represented by the shaded region.

Solution:

(a)

\begin{array}{l} Scale factor \\ = \frac{3}{6 + 3} = \frac{3}{9} = \frac{1}{3} \end{array}

(b)

Area of image = k²× Area of object

\begin{array}{l} 15 = {(\frac{1}{3})}^{2} \times Area of object \\ Area of object = 15 \times 9 = 135 \\ Area of shaded region = 135 - 15 \\ = 120 c m^{2} \end{array}

10.1 Transformations II

Posted on April 16, 2018 by user

10.1 Transformations II

10.1.1 Similarity

Two shapes are similar if

(a) the corresponding angles are equal and

(b) the corresponding sides are proportional.

Example:

Quadrilateral ABCD is similar to quadrilateral JKLM because

\begin{array}{l} ∠ A = ∠ J = 90^{o} \\ ∠ B = ∠ K = 50^{o} \\ ∠ C = ∠ L = 130^{o} \\ ∠ D = ∠ M = 90^{o} \end{array}

(All the corresponding angles are equal.)

\begin{array}{l} \frac{A B}{J K} = \frac{5}{10} = \frac{1}{2} \\ \frac{B C}{K L} = \frac{4}{8} = \frac{1}{2} \\ \frac{C D}{L M} = \frac{2.5}{5} = \frac{1}{2} \\ \frac{A D}{J M} = \frac{3}{6} = \frac{1}{2} \end{array}

(All the corresponding sides are proportional.)

10.1.2 Enlargement

1. Enlargement is a type of transformation where all the points of an object move from a fixed point at a constant ratio.

2. The fixed point is known as the centre of enlargement and the constant ratio is known as the scale factor.

Scale factor = \frac{length of side of image}{length of side of object}

3. The object and the image are similar.

4. If A’ is the image of A under an enlargement with centre O and scale factor k, then

\frac{O A'}{O A} = k

if k > 0, then the image is on the same side of the object.
if k < 0, then the image is on the opposite side of the object.
if –1 < k < 1, then the size of the image is a reduction of the size of the object.

5. Area of image = k² × area of object.