Home › SPM Physics › Archive for Forces and Pressure

Forces and Pressure

3.6.2 Applications of Bernoulli’s Principle

Posted on by

Aeroplane

  1. When a wing in the form of an aerofoil moves in air, the flow of air over the top travels faster and creates a region of low pressure. The flow of air below the wing is slower resulting in a region of higher pressure.
  2. The difference between the pressures at the top and underside of the wing causes a net upward force, called lift, which helps the plane to take-off.

Q & A

Q: Explain how an upthrust is produced when the aeroplane is moving.

A:
  1. When the aeroplane is moving, air flows faster above the wing than below.
  2. Therefore, the air pressure below the wing is higher than above.
  3. The difference in air pressure produces a net force acting upwards.

Q & A

Q: There are slat in front and flaps at the back of the wings of an aeroplane. Describe with the aid of a diagram how the slat and flaps of the wings help in lifting the aeroplane when the aeroplane starts to depart.
A: 
  1. When the aeroplane starts to depart, the slat and flaps are stretched and spread out to increase the surface area of the wings.
  2. This increases the lifting force acting on the aeroplane.

Sports

In some of the sport such as football, a player can make the ball move in a curve path by spinning the ball. This effect can be explained by Bernoulli's Principle.

Insecticide Spray

 

  1. When the plunger is pushed in, the air flows at a high velocity through a nozzle.
  2. The flow of air at high velocity creates a region of low pressure above the metal tube. The higher pressure of the atmospheric air acts on the surface of the liquid insecticide causing it to rise up the metal tube.
  3. The insecticide leaves the top of the metal tube through the nozzle as a fine spray.

Bunsen Burner

  1. When the burner is connected to a gas supply, the gas flows at high velocity through a narrow passage in the burner, creating a region of low pressure.
  2. The outside air, which is at atmospheric pressure, is drawn in and mixes with the gas.
  3. The mixture of gas and air enables the gas to burn completely to produce a clean, hot, and smokeless flame

Carburetor

A carburetor is a device that blends air and fuel for an internal combustion engine. Figure above shows how Bernoulli's principle is applied in a carburetor to mix the air with the fuel.

Q & A

Q: Explain why 2 fast moving boats tend to move closer to each other.

A: 
  1. When the two boats travel at high speed, the stream of fluid (air and water) between the boats flow faster than the other sides of the boats.
  2. This form a low pressure zone in between the boats.
  3. The higher pressure at the other sides of the boat pushes the boats closer to each other.

 

3.6.1 Bernoulli’s Principle

Posted on by

Bernoulli's Principle states that as the speed of a moving fluid (liquid or gas) increases, the pressure within the fluid decreases.


Venturi Effect

The Venturi effect is the fluid pressure that results when an incompressible fluid flows through a constricted section of a pipe.

Experiment 1

Figure above shows that when water flow from left to right, the water level decreases from left to right. This indicates that, the water pressure decreases from left to right.

Explanation:
Liquids flow from places with higher pressure to places with lower pressure.


However, if the experiment is repeated by using a Venturi tube where the diameter at B is made smaller than A and C as in the diagram above, the water level become lowest at B.

Explanation:
The pressure at B is the lowest because the liquid flow the fastest at B. According to Bernoulli's Principle, the faster the water flow, the lower the water pressure.


Experiment 2


Figure above shows some air is blow through a tube from left to right. The water level in the capillary tube increases from left to right.
This indicates that the pressure in the tube decreases from left to right.

Explanation:
Gases flow from places with higher pressure to places with lower pressure.


However, if the tube is replaced by a Venturi tube, the water level become highest at B. This indicates that, the pressure of the air is the lowest at B.

Explanation:
The pressure at B is the lowest because the gas flow the fastest at B. According to Bernoulli's Principle, the faster the gas flow, the lower the gas pressure.

Experiment 1

A paper will be lifted upwards when air is blown rapidly above it.

Explanation:
  1. Air move rapidly above the paper, causes the pressure above the paper to decrease.
  2. Pressure below the paper becomes relatively higher.
  3. Owing to the difference of the pressure, a net force is produced to push the paper upward.

Experiment 2

When air is blown rapidly between the 2 ping pong balls, the 2 balls will move towards each other.

Explanation:
  1. Air move rapidly between the 2 balls, causes the pressure between the 2 balls to decrease.
  2. Pressure at the other side of the balls becomes relatively higher, push the 2 ball close to each other.

Experiment 3

The ping pong ball will not fall when water is allowed to flow through the filter funnel.

Explanation:
  1. Water above the ping pong ball flow rapidly, causes the pressure above the ping pong ball to decrease.
  2. As a result, the pressure below the ping pong ball is relatively higher.
  3. Owing to the difference of the pressure, a net force is produced to push the ping pong ball upward.

 

3.5.3 Appilcations of Archimedes Principle

Posted on by

Plimsoll Line


The Plimsoll line is an imaginary line marking the level at which a ship or boat floats in the water.
It indicates how much load is allowed at different types of water.

Airship


  1. Air ship is filled with helium gas.
  2. Helium gas has density lower than the surrounding air, hence an upthrust which higher than the weight of the airship can be produced and cause the airship float in the air.

Hot Air Balloon


  1. Hot air in the balloon has lower density than the surrounding air.
  2. As a result, when the buoyant force produced is higher than the weight of the balloon, the balloon will start rising up.
  3. The altitude of the balloon can be controlled by varying the temperature of the air in the balloon.

Hydrometers


  1. Hydrometer is used to measure relative density of liquids.
  2. How deep the hydrometer sink into the liquid is affected by the density of the liquid.
  3. The lower the density of the liquid, the deeper the hydrometer will sink.
  4. This is used as the indicator of relative density of a liquid.

Submarine


A submarine use ballask tank to control its movement up and down.
To get submerge, water is pumped into the ballast tank to increase the weight of the submarine.
To surface, the water is pumped out to reduce the weight of the submarine.

Q & A

Q: The diagram shows a picture of a hydrometer. What is the function of the lead shot at the bottom of the hydrometer?

A: 

To lower down the centre of gravity of the hydrometer. The hydrometer will topple if the centre gravity of the hydrometer is above the surface of the liquid.

 

3.5.2 Principle of Floatation

Posted on by
  1. The principle of floatation states that when an object floats in a liquid the buoyant force/upthrust that acts on the object is equal to the weight of the object.
  2. As shown in the figure above, if the weight of the object (W) = upthrust (F), the object is in balance and therefore float on the surface of the fluid.
  3. If the weight of the object > upthrust, the object will sink into the fluid.

Note

  1. Displaced volume of fluid = volume of the object that immerse in the fluid.
  2. If weight of the object > upthrust, the object will sink into the fluid.
  3. If weight of the object = upthrust, the object is in balance and therefore float on the surface of the fluid.

In order to solve the problem related to object immerse in water, it's important to know the all forces acted on the object.

Case 1:


  1. The density of the object is lower than the density of the liquid. The object floats on the surface of the water.
  2. The forces acting on the object is
    1. the weight of the object(W)
    2. the upthrust (F)
Forces are in equilibrium, hence

F = W

Case 2:

  1. The density of the object is greater than the density of the liquid. The object sink to the bottom of the water.
  2. Lying on the bottom of the water, there is a normal reaction acted on the object.
  3. The forces acting on the object is
    1. the weight of the object(W)
    2. the upthrust (F)
    3. Normal reaction (R)
Forces are in equilibrium, hence
F + R = W

Case 3:

  1. The density of the object is greater than the density of the liquid. The object is hold by a string so that it does not sink deeper into the water.
  2. The forces acting on the object is
    1. the weight of the object(W)
    2. the upthrust (F)
    3. Tension of the string (T)
Forces are in equilibrium, hence
F + T = W

Case 4:

  1. The density of the object is lower than the density of the liquid. The object is hold by a string so that it does not move up to the surface of the water.
  2. The forces acting on the object is
    1. the weight of the object(W)
    2. the upthrust (F)
    3. Tension of the string (T)
Forces are in equilibrium, hence

F = W + T

Example 1:



A metal block that has volume of 0.2 m³ is hanging in a water tank as shown in the figure to the left. What is the tension of the string? [ Density of the metal = 8 × 10³ kg/m³, density of water = 1 × 10³ kg/m³]

Answer:
Let,
Tension = T
Weight = W
Upthrust = F

Diagram below shows the 3 forces acted on the block.



The 3 forces are in equilibrium, hence



Example 2:
A wooden sphere of density 0.9 g/cm³ and mass 180 g, is anchored by a string to a lead weight at the bottom of a vessel containing water. If the wooden sphere is completely immersed in water, find the tension in the string.

Answer:
Let's draw the diagram that illustrate the situation:



We need to determine the volume of the displaced water to find the upthrust.
Let the volume of the wooden sphere = V


Let,
Tension = T
Weight = W
Upthrust = F

All the 3 forces are in equilibrium, hence


Example 3:



Figure above shows a copper block rest on the bottom of a vessel filled with water. Given that the volume of the block is 1000cm³. Find the normal reaction acted on the block.
[Density of water = 1000 kg/m³; Density of copper = 3100 kg/m³]

Answer:
Volume of the block, V = 1000cm³ = 0.001m³

Let,
Normal Reaction = R
Weight = W
Upthrust = F


Diagram below shows the 3 forces acted on the block.


All the 3 forces are in equilibrium, hence

 

3.5.1 Archimedes Principle

Posted on by
  1. Archimedes Principle states that when a body is wholly or partially immersed in a fluid it experiences an upthrust equal to the weight of the fluid displaced.
  2. Upthrust/Buoyant force is an upward force exerted by a fluid on an object immersed in it.
  3. Mathematically, we write
F=ρVg
     F = Upthrust/Buoyant Force
     ρ = Density of the liquid
     V = Volume of the displaced liquid
     g = Gravitational field strength



Example 1:
Determine the upthrust acted on the objects immerse in the water below.
a.

b.

c.


Answer:
a. Upthrust = Weight of the displaced water = 15N

b. Upthrust = Weight of the displaced water = 32N

c. Upthrust = Weight of the displaced water = 20N


Example 2:
An iron block which has volume 0.3m³ is immersed in water. Find the upthrust exerted on the block by the water. [Density of water = 1000 kg/m³]

Answer:

Density of water, ρ = 1000 kg/m³
Volume of water, V = 0.3 m³
Gravitational Field Strength, g = 10 N/kg
Upthrust, F = ?


Example 3:

Figure above shows an empty boat floating at rest on water. Given that the mass of the boat is 150kg. Find
  1. the upthrust acting on the boat.
  2. The mass of the water displaced by the boat.
  3. The maximum mass that the boat can load safely if the volume of the boat at the safety level is 3.0 m³.

Answer:
a. According to the principle of flotation, the upthrust is equal to the weight of the boat.

Upthrust,
F = Weight of the boat
= mg = (150)(10) = 1500N

b. According to the Archimedes' Principle, the weight of the water displaced = Upthrust

Weight of the displaced water,
W = mg
(1500) = m(10)
m = 150kg

c.
Maximum weight can be sustained by the boat


Maximum weight of the load
= Maximum weight sustained - Weight of the boat
= 30,000 – 1,500 = 28,500N

Maximum mass of the load
= 28500/10 = 2850 kg


Example 4:

In figure above, a cylinder is immersed in water. If the height of the cylinder is 20cm, the density of the cylinder is 1200kg/m³ and the density of the liquid is 1000 kg/m³, find:
a. The weight of the object
b. The buoyant force

Answer:
a.
Volume of the cylinder, V  = 50 x 20 = 1000cm³ = 0.001m³
Density of the cylinder, ρ = 1200 kg/m³
Gravitational Field Strength, g = 10 N/kg
Weight of the cylinder, W = ?

b.
Volume of the displaced water = 50 x 12 = 600cm³ = 0.0006m³
Density of the water, ρ = 1000 kg/m³
Upthrust, F = ?
 


Example 5
The density and mass of a metal block are 5.0×103 kg m-3 and 4.0kg respectively. Find the upthrust that act on the metal block when it is fully immerse in water.
[ Density of water = 1000 kgm-3 ]

Answer:
In order to find the upthrust, we need to find the volume of the water displaced. Since the block is fully immersed in water, hence the volume of the water displaced = volume of the block.

Volume of the block,


Upthrust acted on the block,

 

3.4.3 Hydraulic Braking System and Hydraulic Jack

Posted on by

Hydraulic Brake

In most vehicle, hydraulic system is used in the braking system, as shown in the figure below.


Usually, a disc brake is used in the front wheel of a car while a drum brake is used in the back wheel of a car.

Working Mechanism of Hydraulic Brake

  1. When the brake pedal is pressed, the piston of the master cylinder applies a pressure on the brake fluid.
  2. This pressure is transmitted uniformly to each cylinder at the wheel, cause the pistons at the wheels to push the brake shoes to press against the surface of the brake.
  3. The friction between the brakes and brake shoes causes the vehicle to slow down and stop.

Q & A

Q: Why is it dangerous if air bubble is trapped in the brake fluid of a braking system.

 A: 
  1. If air bubble is present in the fluid, the fluid become compressible. 
  2. This may prevent pressure transmits through the fluid and hence causing ineffective braking effect.

Q & A

Q: Why oil but not water is used as the hydraulic fluid in a hydraulic brake system?

 A:
  1. Because the boiling point of oil is much higher than water. This can prevent the hydraulic fluid from boiling when the brake is very hot. 
  2. Water may cause rusting in the part of the braking system

Hydraulic Jack

Working mechanism of a hydraulic jack.

  1. When the handle is pressed down, valve A is closed whereas valve B is opened. The hydraulic fluid is forced into the large cylinder and hence pushes the piston moving upward.

  3. When the handle is raised, valve B will be closed while vale A will be opened. Hydraulic fluid from the buffer tank will be suck into the small cylinder.

  5. This process is repeated until the load is sufficiently lifted up.
  6. The large piston can be lowered down by releasing the hydraulic fluid back to the buffer tank through the release vale.

 

3.4.2 Hydraulic System

Posted on by

  1. A hydraulic system applies Pascal's principle in its working mechanism. It can be used as a force multiplier.
  2. In this hydraulic system, a small force, Fl is applied to the small piston X results in a large force, F2 at the large piston Y. The pressure, due to the force, F1, is transmitted by the liquid to the large piston.
  3. According to Pascal’s principle,


Change of Oil Level in a Hydraulic System


In the diagram to the left, when piston-X is pressed down, piston-Y will be push up. The change of the piston levels of the 2 pistons is given by the following equation:

Example 1:
In a hydraulic system the large piston has a cross-sectional area A2 = 200 cm² and the small piston has cross-sectional area A1 = 5 cm². If a force of 250 N is applied to the small piston, what is
a. the pressure exerted on the small piston
b. the force F, produced on the large piston?

Answer:

a. Pressure exerted on the small piston

b. Pressure exerted on the large piston = Pressure exerted on the small piston



Example 2:

A hydraulic lift is to be used to lift a truck masses 5000 kg. If the diameter of the small piston and large piston of the lift is 5cm and 1 m respectively,

a. what gauge pressure in Pa must be applied to the oil?
b. What is the magnitude of the force required on the small piston to lift the truck?

Answer:
a. Weight of the truck,
W = mg
W = (5000)(10) = 50,000N

Area of the big piston


Pressure of the oil


b.
Area of the small piston


According to the Pascal's Principle,


Example 3:

Figure above shows a hydraulic system. The area of surface X is 5 cm² and the area of surface Y is 100 cm². Piston X has been pushed down 10cm. what is the change of liquid level, h, at Piston Y?

Answer:

Distance move by the piston-X, h1 = 10cm
Distance move by the piston-Y, h2 = h
Area of piston-X, A1 = 5 cm²
Area of Piston-Y, A2 = 100 cm²


 

3.4.1 Pascal’s Principle

Posted on by
  1. Pascal's principle states that in a confined fluid, an externally applied pressure is transmitted uniformly in all directions.
  2. Pascal's principle is also known as the principle of transmission of pressure in a liquid.

Q & A

Q: Suggest an experiment to prove Pascal’s Principle.

A:
  1. When the plunger is pushed in, the water squirts equally from all the holes.
  2. This shows that the pressure applied to the plunger has been transmitted uniformly throughout the water.

 

3.3.5 Applications of Atmospheric Pressure

Posted on by

Syringe

  1. When the piston is pulled up, the atmospheric pressure inside the cylinder will decrease.
  2. The atmospheric pressure outside pushes the liquid up into the syringe.

Lift Pump

Siphon

Working Mechanism of Siphon

Sucker Hook


When the sucker is pressed into place, the air inside is forced out. As a result, the pressure inside the sucker become very low. The sucker is then held in position by the high atmospheric
pressure on the outside surface.

Straw


When a person sucks through the straw, the pressure in the straw become low. The atmospheric pressure outside which is higher will force the water into the straw and consequently into the mouth.

Rubber Sucker

Vacuum Cleaner


 

 

 

3.3.4 Measuring Atmospheric Pressure

Posted on by
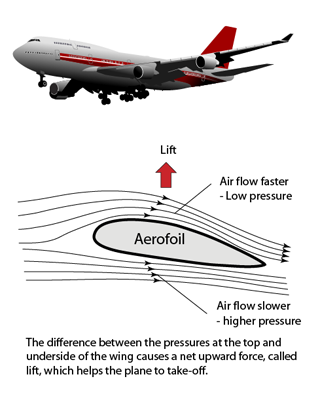
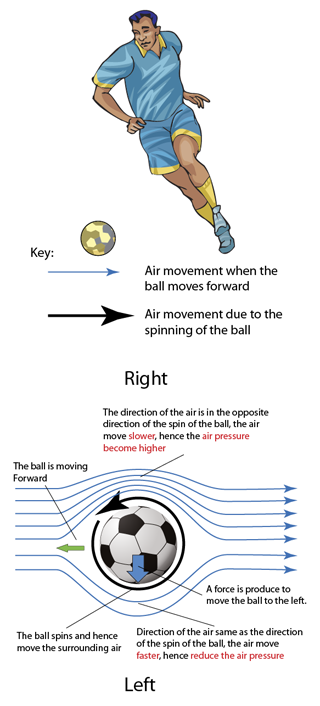
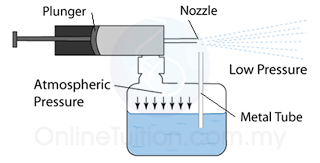
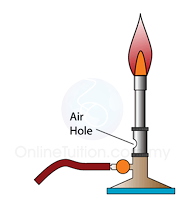
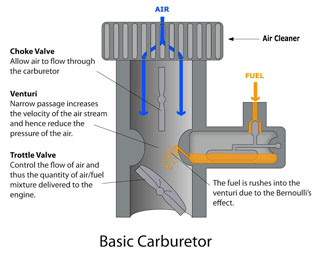
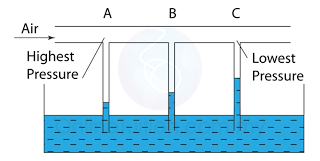
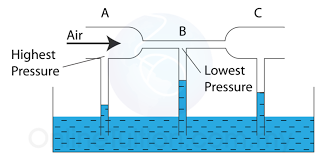
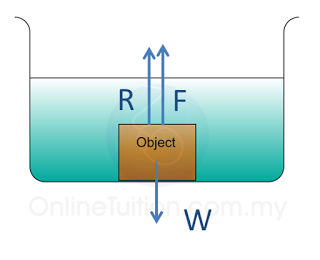
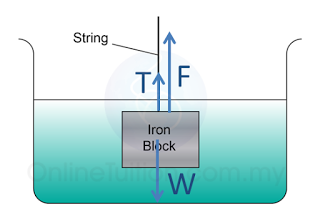
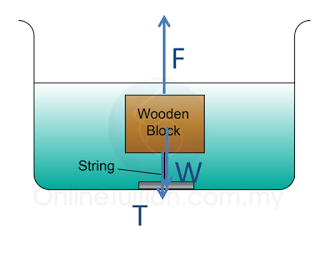
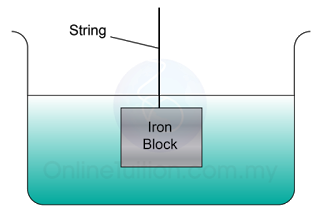
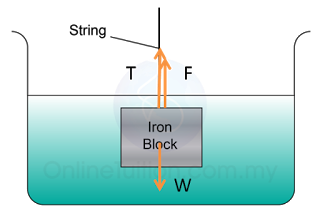
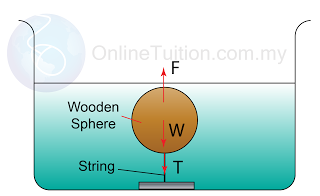
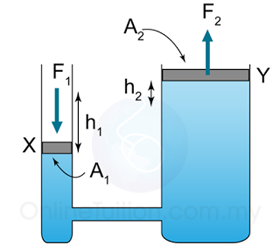
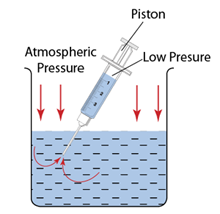
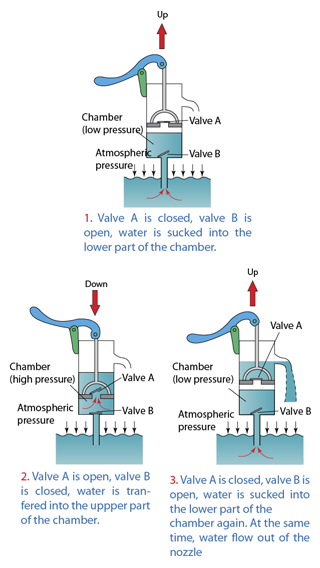
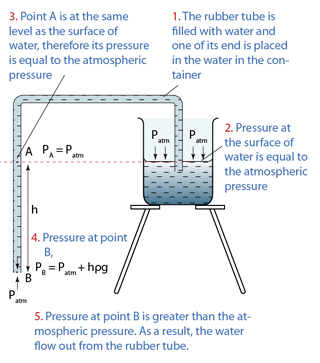
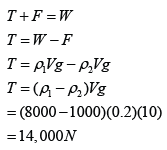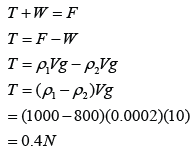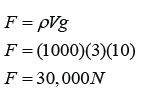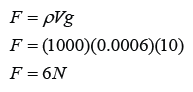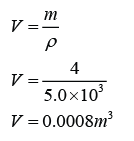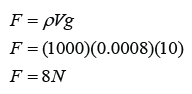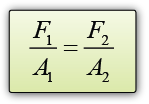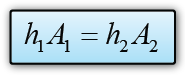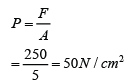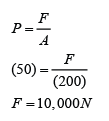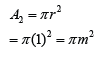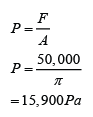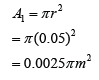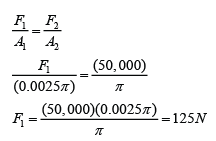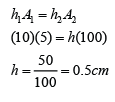  1. Atmospheric pressure can be measured by either
    1. a simple barometer,
    2. a Fortin barometer
    3. an Aneroid barometer.
  2. In SPM, most of the questions asked are related to the simple barometer.
  3. For Fortin barometer and Aneroid barometer, you only need to know their working principle.

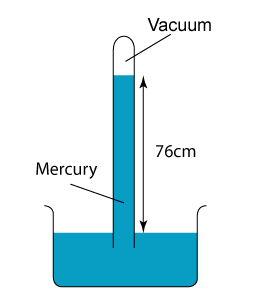
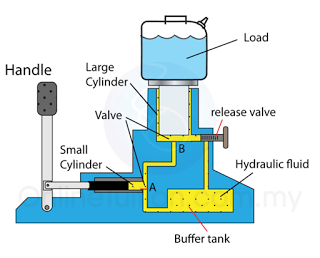
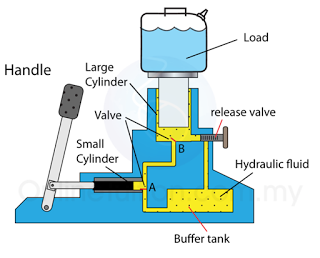
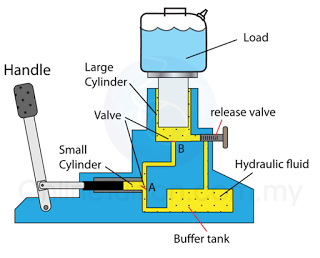
Simple Mercury Barometer

Fortin Barometer.
This image is created by Edal Anton Lefterov and shared under the Creative Commons Attribution-Share Alike 3.0 Unported license.



Aneroid Barometer

Simple Barometer

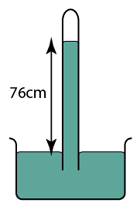
  1. Atmospheric pressure can be measured by a simple barometer.
  2. A thick glass tube (at least 1m long) is filled with mercury completely.
  3. The open end of the tube is covered with a finger and inverted in a trough of mercury.
  4. The height of the mercury is proportional to the atmospheric pressure.

Using Simple Mercury Barometer


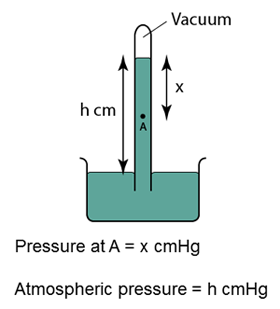
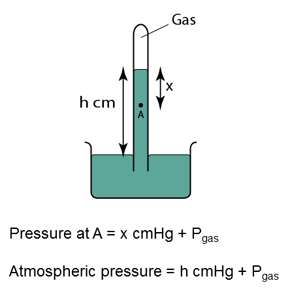
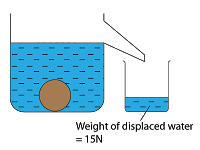
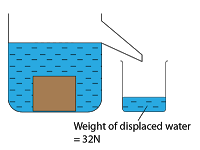
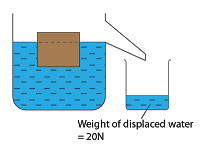
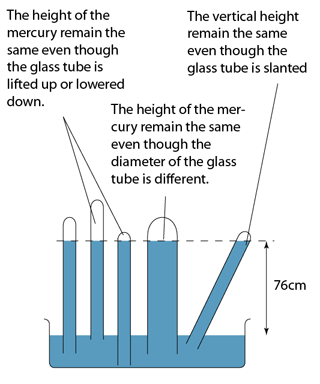
Characteristics of the Mercury Barometer



Q and A
Q: Barometer is usually made up of mercury. Explain why is it not practical to have a water barometer?

A: 
  1. The atmospheric pressure is about 10 meter water, which means it can push the water up to 10 meter height. 
  2. Therefore a water barometer must be at least 10 meter long. 
  3. This is not practical because the glass tube of the barometer may be broken or topple easily. It is also difficult to keep or move such a long tube.

Fortin Barometer

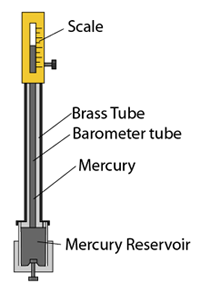
  1. The Fortin barometer is an improved version of the simple mercury barometer.
  2. The barometer tube is encased in a brass frame. This enables it to be carried around easily.
  3. Vernier scale is used for taking reading for extra accuracy.

Q & A

Q: What is the advantages of a Fortin Barometer over a Simple mercury barometer?

A:
  1. Easy to be carried around.
  2. More accurate.

Anaroid Barometer


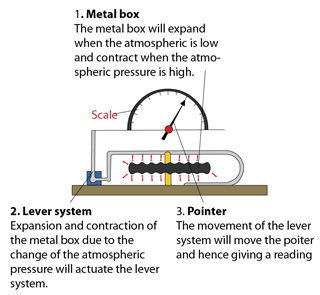
The anaroid barometer is usually used to measure altitude, which is named as altimeter.

Q & A

Q: Explain why the barometer can be used to measure altitude.

A:
The atmospheric pressure is inversely proportional to the altitude. The altitude can be determined from the atmospheric pressure.


Q & A

Q: Why a simple barometer is not suitable to be used to measure altitude?

A:
  1. Hard to be carried
  2. Mercury is volatile. It can evaporate easily
  3. Mercury is poisonous

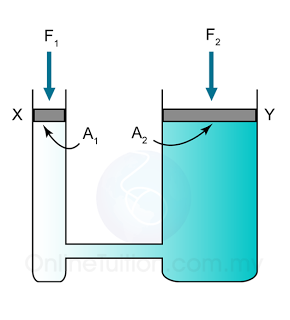
Converting the Unit from cmHg to Pa

Pressure in unit cmHg can be converted to Pa by using the formula

P = hρg

Example 1:

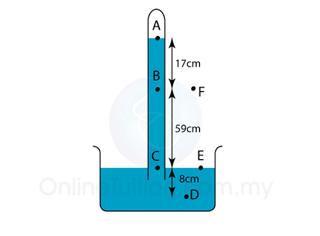
Find the pressure at point A, B, C, D, D, E and F in the unit of cmHg and Pa. [Density of mercury = 13600 kg/m³]

Answer:
Pressure in unit cmHg  Pressure in unit Pa 
PA = 0

PB = 17 cmHg

PC = 17 + 59 = 76 cmHg

PD = 76 + 8 = 84 cmHg

PE = 76 cmHg

PF = 76 cmHg		 PA = 0

PB = hρg = (0.17)(13600)(10) = 23,120 Pa

PC = hρg = (0.76)(13600)(10) = 103,360 Pa

PD = hρg = (0.84)(13600)(10) = 114,240 Pa

PE = hρg = (0.76)(13600)(10) = 103,360 Pa

PF = hρg = (0.76)(13600)(10) = 103,360 Pa


Example 2:

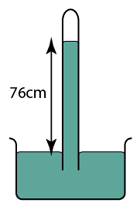
Figure above shows a simple mercury barometer. What is the value of the atmospheric pressure shown by the barometer? [Density of mercury = 13600 kg/m³]

Answer:
Atmospheric Pressure,

P = 76 cmHg

or

P = hρg = (0.76)(13600)(10) = 103360 Pa


Example 3:

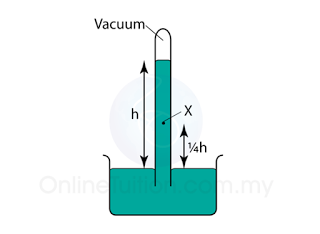
In above, the height of a mercury barometer is h when the atmospheric pressure is 101 000 Pa.
What is the pressure at X?

Answer:
Atmospheric Pressure,
Patm = h cmHg = 101 000 Pa

Pressure at X,
PX = (h - ¼h) = ¾h cmHg

PX = ¾ x 101 000 = 75 750 Pa


Example 4:

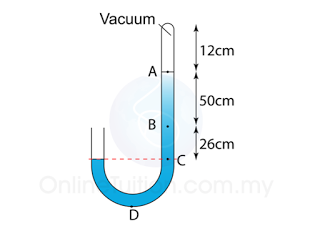
Figure above shows a mercury barometer whereby the atmospheric pressure is 760 mm Hg on a particular day. Determine the pressure at point
a. A,
b. B,
c. C.
[Density of Mercury = 13 600 kg/m³]
Answer:
a.
PA = 0

b.
PB = 50 cmHg

or

PB = hρg = (0.50)(13600)(10) = 68 000 Pa

c.
PC = 76 cmHg

or

PC = hρg = (0.76)(13600)(10) = 103 360 Pa


Example 5:

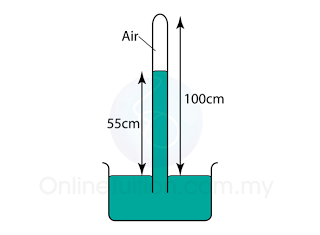
Figure above shows a simple barometer, with some air trapped in the tube. Given that the atmospheric pressure is equal to 101000 Pa, find the pressure of the trapped gas. [Density of Mercury = 13 600 kg/m³]

Answer:
Pressure of the air = Pair
Atmospheric pressure = Patm

Pair + 55 cmHg = Patm

Pair
= Patm - 55 cmHg
= 101 000 - (0.55)(13 600)(10)
= 101 000 - 74 800
= 26 200 Pa


Example 6:
If the atmospheric pressure in a housing area is 100 000 Pa, what is the magnitude of the force exerted by the atmospheric gas on a flat horizontal roof of dimensions 5m × 4m?

Answer:
Area of the roof = 5 x 4 = 20 m²

Force acted on the roof

F = PA
F = (100 000)(20)
F = 2,000,000 N


Example 7:


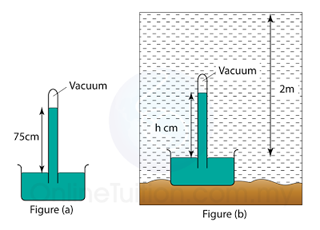
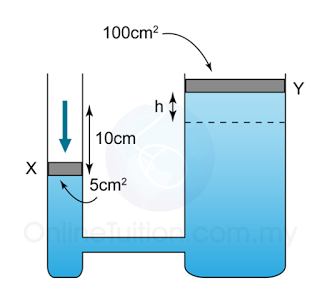
Figure(a) above shows the vertical height of mercury in a mercury barometer in a laboratory. Figure(b) shows the mercury barometer in water at a depth of 2.0 m.

Find  the vertical height (h) of the mercury in the barometer in the water. Given that the pressure at a depth of 10 m from the water surface is 75 cmHg. [Density of water = 1000 kg/m³, Density of mercury = 13 600 kg/m³]

Answer:
Atmospheric pressure,
Patm = 75 cmHg

Pressure caused by the water,
Pwater = 2/10 x 75 = 15cmHg

Pressure in 2m under water
= 75 + 15 = 90 cmHg

Vertical height of the mercury = 90cm