Home › SPM Physics › Archive for Heat › Page 2

Heat

4.3.1 Latent Heat

Posted on by
  1. When a solid melt, heat is absorbed but the temperature remains constant.
  2. When a liquid is boiling, heat is also absorbed but the temperature again remains constant.
  3. The heat absorbed or given out at constant temperature during the change of phase is known as latent heat.
  4. The heat energy that releases during condensation or boiling is called the latent heat of vaporization.
  5. The heat energy that releases during freezing or melting is called the latent heat of fusion.
The latent heat is the heat absorbed or given out at constant temperature during the change of state of matter.

 

4.2.3 Applications of Specific Heat Capacity

Posted on by

Cooking Pot

  1. Different part of a cooking pot are made up of different material.
  2. The base of a cooking pot is usually made up of copper because
    1. copper has low specific heat capacity so that it need less heat to raise up the temperature.
    2. copper is a good heat conductor.
    3. copper has high density. The heavier base can make the pot become more stable. 
  3. The handles of cooking pot is usually made up of plastic or wood because
    1. plastic and wood have high specific heat capacity. Their temperature won’t become too high even it absorbs large amount of heat.
    2. plastic and wood are good heat insulator.
    3. plastic and wood have low density hence they do not add much to the total weight of the pot.
  4. The body of the cooking pot is usually made up of stainless steel because
    1. steel has low specific heat capacity and hence need need less heat to raise up the temperature.
    2. steel does not react chemically with the food.

Car Engine


  1. Water is used to cool down the car engine.
  2. Water is used as the cooling agent in the car cooling system because 
    1. it has high specific heat capacity. It can absorb a large amount of heat without a high increase in temperature.
    2. it is cheap and can be obtained easily.
  3. Water is pumped through the channels in the engine block to absorb heat.
  4. The hot water flows to the radiator and is cooled by the air flows through the fins of the radiator.
  5. The cool water flows back to the engine again to capture more heat and this cycle is repeated continuously.

Applications of Specific Heat Capacity - Factories with Low Ceiling

  1. Some factories without large machinery are constructed with low ceilings to reduce the volume of air inside the building. 
  2. The smaller mass of air will have a smaller heat capacity.
  3. As a result, less heat needs to be removed to reduce the temperature of the air.
  4. This will then reduce the air conditioning costs for the factory.

Thermal Radiator

  1. Thermal radiators are always used in cold country to warm the house.
  2. Hot water is made to flow through a radiator. The heat given out from the radiator is then warm the air of the house.
  3. The cold water is then flows back to the water tank. This process is repeated continuously.
  4. Water is used in the radiator because it has high specific heat capacity.

Sea Breeze

  1. Land has lower heat capacity than sea water. Therefore, in day time, the temperature of the land increases faster than the sea.
  2. Hot air (lower density) above the land rises. Cooler air from the sea flows towards land and hence produces sea breeze.

Land Breeze

  1. Land has lower heat capacity than sea water. During night time, the temperature of the land drops faster than the sea.
  2. Hot air (lower density) above the sea rises. Cooler air from the land blows towards sea and hence produces land breeze.

Moderate Climate

  1. Places with the presence of lakes, sea and ocean may have more moderate climate.
  2. This is because, water has large specific heat capacity.
  3. During daytime when it is hot, the water from the lake/sea absorbs heat from the surroundings. This helps to reduce the temperature of the surroundings.
  4. During night-time, the water releases the heat absorbed during daytime, and hence prevents the temperature from dropping too much.
  5. As such, palcess near a large mass of water will have a smaller range of temperatures and hence a more moderate climate condition.

 

4.2.2 Specific Heat Capacity

Posted on by
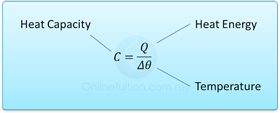
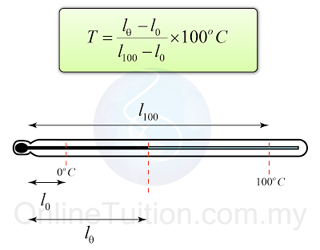
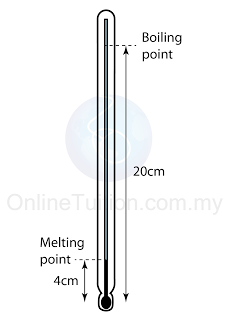
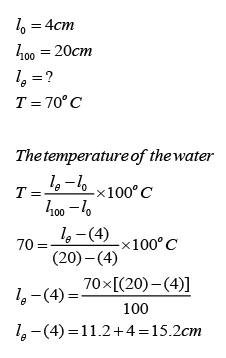
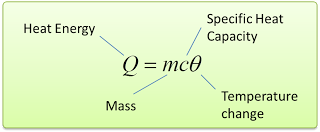
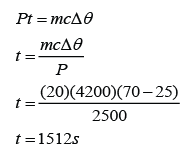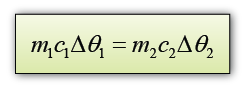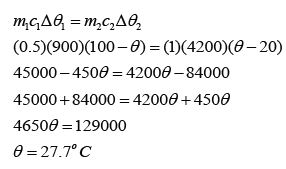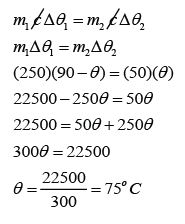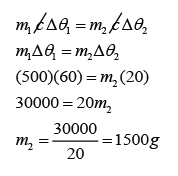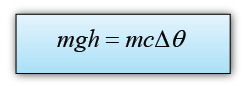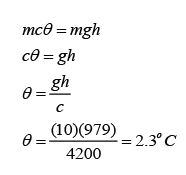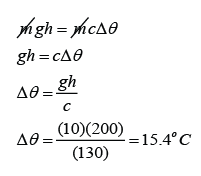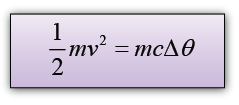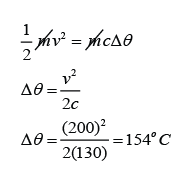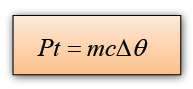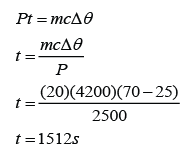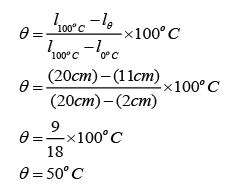Specific heat capacity is defined as the amount of heat required to change the temperature of 1 kg of a substance by 1°C.
  1. Specific heat capacity is a physical quantity used to compare the heat capacity of a given material of the same mass.
  2. It is the measure of how much energy can be store in 1 kg of mass of a substance.
  3. Specific heat capacity is defined as the amount of heat required to change the temperature of 1 kg of a substance by 1°C.
    Mathematically, we write this as c = Q/mθ [Q = heat, c = specific heat capacity, m = mass, θ = change of temperature]
  4. The SI unit of specific heat capacity is J/kg/°C.
  5. For example, the specific heat capacity of water is 4200 J/kg/°C means 4200J of heat energy is needed to change the temperature of 1kg water by 1°C.
  6. The amount of heat transferred in an object when temperature change can be calculated by using the following equation.

Example 1:
How much heat energy is required to change 2 kg of ice at 0°C into water at 20°C? [Specific latent heat of fusion of water = 334 000 J/kg; specific heat capacity of water = 4200 J/(kg K).]

Answer:
m = 2kg
Specific latent heat of fusion of water, L = 334 000 J/kg
specific heat capacity of water = 4200 J/(kg K)

Energy needed to melt 2kg of ice,
Q1 = mL = (2)(334000) = 668000J

Energy needed to change the temperature from 0°C to °C.
Q2 = mcθ  = (2)(4200)(20 - 0) = 168000J

Total energy needed = Q1 + Q2 = 668000 + 168000 = 836000J


Example 2:
Starting at 20°C, how much heat is required to heat 0.3 kg of aluminum to its melting point and then to convert it all to liquid? [Specific heat capacity of aluminium = 900J kg-1 °C-1; Specific latent heat of aluminium = 321,000 Jkg-1, Melting point of aluminium = 660°C]

Answer:
m = 0.3kg
Specific latent heat of fusion of aluminium, L = 321 000 J/kg
specific heat capacity of aluminium = 900 J/(kg K)

Energy needed to increase the temperature from 20°C to 660°C
Q1 = mcθ  = (0.3)(900)(660 - 20) = 172,800J

Energy needed to melt 0.3kg of aluminium,
Q2 = mL = (0.3)(321000) = 96,300J

Total energy needed = Q1 + Q2 = 172,800 + 96,300 = 269,100J



Example 3:
How much heat must be removed by a refrigerator from 2 kg of water at 70 °C to convert it to ice cubes at -11°C? [Specific heat capacity of water = 4200J kg-1 °C-1; Specific latent heat of fusion of ice = 334,000 Jkg-1, specific heat capacity of ice = 2100 J/(kg K)]

Answer:
m = 2kg
Specific latent heat of fusion of water, L = 334,000 J/kg
Specific heat capacity of water, cw = 4,200 J/(kg K)
Specific heat capacity of ice, ci = 2,100 J/(kg K)

Energy to be removed to reduce the temperature from 70°C to 0°C (Freezing point of water)
Q1 = mcθ  = (2)(4200)(70 - 0) = 588,000J

Energy needed to freeze 2kg of water,
Q2 = mL = (2)(334,000) = 668,000J

Energy to be removed to reduce the temperature from 0°C to -11°C
Q3 = mcθ  = (2)(2100)(0 - (-11)) = 46,200J

Total energy needed = Q1 + Q2  + Q3 = 588,000 + 668,000 = 46,200J = 1,302,200J


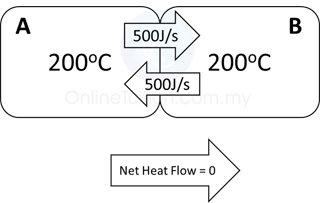
When 2 objects/substances are in thermal contact, there will be a net flow of thermal energy from the object/substance with higher temperature to the object/substance with lower temperature. If we assume there is no thermal energy loss to the surrounding

Thermal energy loss = Thermal energy gain

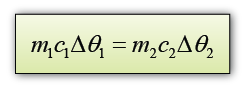
Example 1:

A 0.5 kg block of aluminium at a temperature of 100 °C is placed in 1.0 kg of water at 20 °C. Assuming that no thermal energy is lost to the surroundings, what will be the final temperature of the aluminium and water when they come to the same temperature? [The specific heat capacity of water is 4200 Jkg-1K-1 and The specific heat capacity of aluminium is 900 Jkg-1K-1]

Answer:
Let's say the final temperature for both aluminium block and water = θ

For aluminium,
m1 = 0.5 kg
c1 = 900 Jkg-1K-1
∆θ1 = 100 °C - θ

For water,
m2 = 1.0kg
c2 = 4200 Jkg-1K-1
∆θ2 = θ - 20 °C


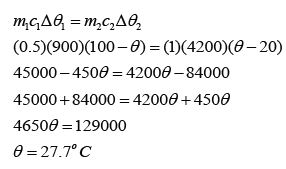
Example 2:
What will be the final temperature if 50 g of water at 0 °C is added to 250 g of water at 90 °C?

Answer:
For water at 90 °C,
m1 = 250g
c1 = c2 = c
∆θ1 = 90 °C - θ

For water at 0 °C,
m2 = 50g
∆θ2 = θ - 0 °C = θ


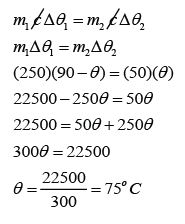
Example 3:
How much water at 10 °C is needed to cool 500 g of water at 90 °C down to 30 °C?

Answer:
For water at 90 °C,
m1 = 500g
c1 = c2 = c
∆θ1 = 90 - 30 = 60 °C

For water at 10 °C,
m2 = ?
∆θ2 = 30 - 10 = 20 °C




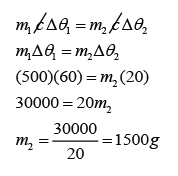
Conversion of Gravitational Energy to Thermal Energy

Example 1:
The Angel Falls in Venezuela is 979 m in height. Calculate the rise in temperature of the water when the water fall from the top to the bottom. (Assume that all the potential energy loss of the water is converted to heat) [The specific heat capacity of water is 4200Jkg-1K-1]

Answer:
The potential energy loss = mgh
The thermal energy gain of the water = mcθ

We assume that all the potential energy loss of the water is converted to heat, hence


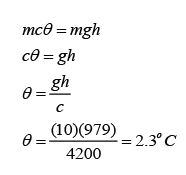
Example 2:
A mass m of lead shot is placed at the bottom of a vertical cardboard cylinder. The cylinder is 1.0 m long and closed at both ends. The cylinder is suddenly inverted so that the shot falls 1.0 m. By how much will the temperature of the shot increase if this process is repeated 200 times? Assume no heat loss to the surrounding. [The specific heat capacity of lead is 130Jkg-1K-1]

Answer:
Distance fall, h = 200 x 1m = 200m
Gratational field strength, g = 10 m/s²


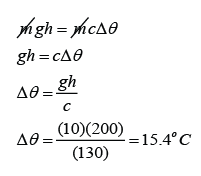
Conversion of Kinetic Energy to Thermal Energy

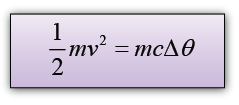
Example 3:
A 2.5g lead bullet is moving at 200 m/s when it strikes a wooden block and is brought to rest. If all the kinetic energy  is transferred to thermal energy in the bullet, find the rise in temperature of the bullet. [The specific heat capacity of lead is 130Jkg-1K-1]

Answer:
v = 200m/s


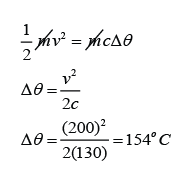
Conversion of Electrical Energy to Thermal Energy

Electrical energy, E = Pt
If we assume that all the electrical energy convert into thermal energy, then

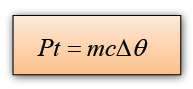
Example 4:
An electric heater supplies 2.5 kW of power in the form of heat to a tank of 0.02m³ of water. How long will it take to heat the water from 25 °C to 70 °C? Assume heat losses to the surroundings to be negligible. [Density of water = 1000kg/m³; The specific heat capacity of water is 4200Jkg-1K-1]

Answer:
P = 2500W
m = (0.02)(1000)=20kg
c = 4200Jkg-1K-1


 

4.2.1 Heat Capacity

Posted on by
  1. Heat capacity is the measure of the ability of an object to store heat as its temperature changes.
  2. It is the measure of the amount of heat required to change the temperature of an object by 1°C.
  3. The SI unit of heat capacity is joules per Kelvin (J/K).
  4. The heat capacity of an object depends on the type of the material the object is made and also the mass of the object. An object with larger mass has higher heat capacity.
  5. An object with higher heat capacity need more heat supplied to change 1 unit of the temperature.

 

4.1.4 Temperature Scale

Posted on by

Calibrating a Thermometer

  1. To calibrate a thermometer means to put the correct mark of reading at the correct place so that other temperature can be deduced from these marks. 
  2. To do this, two extreme points are chosen to mark its scale and these points must be able to be reproduced accurately.
  3. Usually, we take the steam point of pure water as 100°C and the ice point of water as 0°C.
    To calibrate a thermometer, the ice point of water is usually taken to be 0°C  
    To calibrate a thermometer, the steam point is taken to be 100°C

  4. After determining the position of the ice point and steam point, the temperature of an object can be determined by using the formula:

Example 1:
The length of the mercury column in a non-calibrated mercury thermometer is 2cm when its bulb is immerse in melting ice and 20cm when the bulb is in steam above boiling water. What would the temperature be is the length of the mercury column is 11cm?

Answer:
l0°C = 2cm
l100°C = 20cm
lθ = 11cm




Example 2:
The length of the alcohol column in a thermometer is 2.5cm and 17.5cm when the thermometer is dipped into a melting ice and a boiling water respectively. Find the distance between every 10°C of the scale on the thermometer.

Answer:
Distance for 100oC = 17.5cm – 2.5 cm = 15.0cm

Distance for 10oC = 17.5cm – 2.5 cm
= 15.0cm ÷ 10 = 1.50 cm


Example 3:


Figure above shows the length of the mercury thread of a thermometer at melting point and boiling point of water. What is the length of the mercury thread when the thermometer is dipped into a hot liquid of temperature 70°C?

Answer:


Absolute Zero and the Kelvin Temperature Scale

  1. Absolute temperature is the temperature measured in Kelvin scale, which it is a temperature reading made relative to absolute zero.
  2. We can convert a temperature in °C to absolute temperature by adding 273 to the temperature.
    For example:
    25°C = 273 + 25 = 298 K
    100°C = 273 + 100 = 373 K
  3. Absolute zero is the temperature where thermal energy is at minimum. It is 0 on the Kelvin scale and -273 on the Celsius scale.

 

4.1.3 Thermometer

Posted on by

Liquid in Glass Thermometer

  1. Liquid in glass thermometer works on the principle that liquid expands as the temperature increases and contracts as the temperature decreases.
  2. The most commonly used liquids in such thermometers are
    1. Mercury
    2. Alcohol

Q & A

Q: State the advantageous and disadvantageous of using mercury as the liquid in a liquid in glass thermometer.

A:
Advantageous:
  1. Doesn’t wet the wall of the capillary tube.
  2. Can be seen easily
  3. Expand uniformly when heated
  4. Good heat conductor

Disadvantageous:
  1. Freezing point = -39°C. Not suitable to measure temperature lower than -39°C.
  2. Poisonous
  3. Expensive

Q & A

Q: State the advantageous and disadvantageous of using alcohol as the liquid in a liquid in glass thermometer.

A:
Advantageous:
  1. Freezing point = -115°C. Suitable for measuring low temperature.
  2. Expands greater than mercury.

Disadvantageous:
  1. Transparent. Difficult to be seen. Need to be coloured.
  2. Always cling the wall of the capillary tube.
  3. Has tendency to break the tube at high temperature.


Q & A

Q: State the characteristics of the liquid used in a liquid in glass thermometer.

A:
  1. Easily visible
  2. Good conductor of heat
  3. Expand and contract rapidly over a wide range of temperature
  4. Does not cling to the wall of the capillary tube of the thermometer.

Q & A

Q: State and explain how the sensitivity of a liquid in glass thermometer can be increased.
A:
  1. The sensitivity of a mercury thermometer can be increased by using a smaller mercury bulb, thinner wall and smaller bore.
  2. A smaller bulb contains less mercury and hence absorbs heat in shorter time. As a result it can response faster to temperature change.
  3. A glass bulb with thinner wall can transfer heat to the bulb easier. Therefore, the thermometer can response quickly to small changes of temperature near the surrounding.
  4. Capillary with narrow bore produces a greater change in the length of the mercury column. Therefore a small change in temperature can be detected easily.

 

4.1.2 Thermal Equilibrium

Posted on by
  1. Two objects are in thermal contact when heat energy can be transferred between them.
  2. Two objects are in thermal equilibrium when there is no net flow of heat between two objects that are in thermal equilibrium.
  3. Two objects in thermal equilibrium have the same temperature.
Example:
Figure below shows 2 blocks in thermal contact with each other. Initially, the temperature of the 2 blocks are different, and there is a net flow of thermal energy from higher temperature to lower temperature.

After some time, thermal equilibrium achieved, where the temperature of the 2 blocks become the same, and there is no net flow of thermal energy between the 2 blocks.

Before
  1. Initially, the temperature of block A is higher than block B.
  2. The rate of thermal energy transfer is higher from block A to the block B (1000J/s).
  3. There is also thermal energy transfer from the block B to block A, but with lower rate (only at 200J/s).
  4. Therefore, there is a net heat flow of thermal energy from the block A to block B.
  5. As a result, the temperature of block A decreases whereas the temperature of B increases.
After
  1. Thermal Equilibrium Achieved. 
  2. The temperature of the 2 blocks become the same.
  3. Heat flow is still goes on between the blocks.
  4. However, the rate of flow of heat are equal in both direction. As a result, the net heat flow is equal to 0.

Objective Question:
2 iron blocks, P and Q are in thermal contact. The initial temperature of P and Q are 10°C and 50°C respectively. Which of the followings is true when P and Q are at thermal equilibrium.
  1. The temperature of P will be higher than the temperature of Q.
  2. The net flow of heat between P and Q is zero
  3. The temperature of P and Q will be lower than 50°C but higher than 10°C.
  4. There is no heat flow between the 2 blocks.

 Answer:
The correct answer is II and III.

Answer I. is incorrect.
When 2 objects achieve thermal equilibrium, the temperature of the 2 objects will be the same.

Answer II. is correct.
When 2 objects achieve thermal equilibrium, the rate of flow of heat is equal, hence there is no net flow of heat between the 2 objects.

Answer III. is correct.
When P and Q are in thermal contact, the temperature of P (10°C)will increase whereas the temperature of Q (50°C) will decrease. When P and Q are in thermal equilibrium the temperature of P and Q will be the same and the temperature will be higher than 10°C but lower than 50°C.

Answer IV. is incorrect.
In thermal equilibrium, there is still heat flow between P and Q.

However, the rate of the heat flow is equal in both direction (P to Q and Q to P), hence the net flow of heat between P and Q is zero.

Applications of Thermal Equilibrium

Oven

  1. When food such as meat or cake is put in the oven, the heat of the oven is transferred into the food.
  2. This process will continue until the food is in thermal equilibrium with the air in the oven.
  3. This happen when the temperature of the food is equal to the temperature of the air in the oven.

Refrigerator

  1. When food is put in the refrigerator, the heat from the food is transferred into the air of the refrigerator.
  2. This process is continued until the temperature of the food  equal to the temperature of the air in the refrigerator, when thermal equilibrium is reached between the food and the refrigerator.

Thermometer

  1. Thermometer is placed in contact with the patient’s body.
  2. If both the body temperature of the patient and that of the mercury (or alcohol) in the clinical thermometer have reached thermal equilibrium, then the temperature of the thermometer is the same as the body temperature, hence the reading of the thermometer shows the body temperature of the patient.

 

4.1.1 Heat and Temperature

Posted on by
  1. Heat is the flow of thermal energy.
  2. Temperature is a measure of the average kinetic energy which each molecule of an object possesses.

Thermal Energy and Heat

  1. Thermal energy is a measure of the sum of kinetic and potential energy in all the molecules or atoms in an object.
  2. The SI unit of thermal energy is Joule, J.
  3. Heat is the flow of thermal energy, from a hotter body to a colder one.

Temperature

  1. Temperature is a physical quantity which measures the degree of hotness of an object.
  2. Temperature is a measure of the average kinetic energy which each molecule of an object possesses.
  3. One object is at a higher temperature than another if the average kinetic energy of each of its molecules is greater. 
  4. The SI unit of temperature is Kelvin, K.

Differences between Thermal Energy and Temperature

Thermal Energy
Temperature
A form of Energy Degree of hotness of an object.
Unit: Joule (J) Unit: Kelvin (K)/ Degree Celsius (oC)
Sum of the kinetic energy and potential energy of the particles. Average kinetic energy of the particles.
Derived quantity Base quantity