1. In figure (a) above, the light ray is refracted away from the normal when moving from denser medium to less dense medium.
2. Figure (b) shows that, at a specific angle, the light ray is refracted 90o from the normal. It is refracted so much that it is only just able to leave the water. In such condition, the incident angle is called the critical angle.
3. The critical angle is the angle of incident in an optically denser medium for which the angle of refraction is 90°.
4. In figure (c), the light ray strikes the surface at an angle of incidence greater than c. There is no refracted ray; the surface of the water acts like a perfect mirror, and the ray is said to have been totally internally reflected.

 

 

1. The bending of light can give you a false impression of depth.
2. Figure to the left shows two rays of light leaving a point on the bottom of a swimming pool.
3. The rays are refracted as they leave the water. To the observer, the rays seem to come from a higher position, and the bottom looks closer to the surface than it really is.
4. The real depth of the water and its apparent depth are marked on the diagram. These are related to the refractive index of the water by the following equation:

      or    

Summary:

Refractive index

 

 

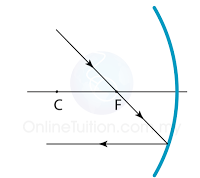
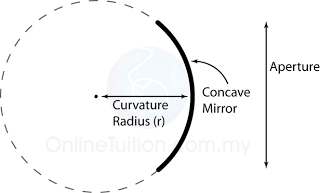
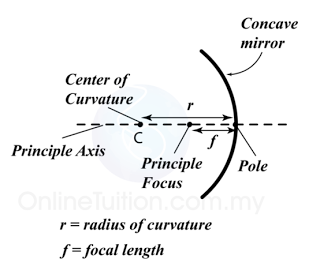
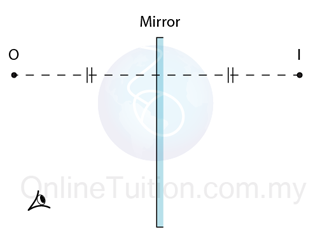
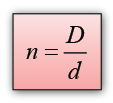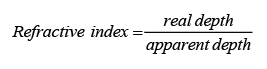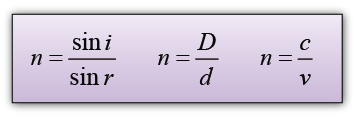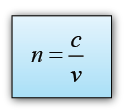Bending of Object in a Glass

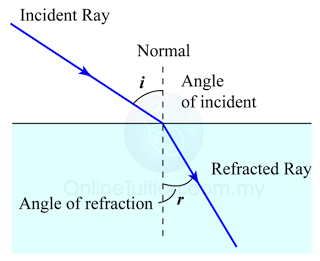
A straw in a glass with water looks bended or broken. This is due to refraction of light

Shallower Swimming Pool

A swimming pool appears shallower than it actual is. This is because the light from the pool is refracted away from the normal when moving from water to the air.

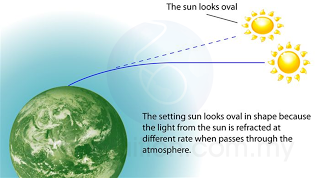
Atmospheric Refraction and Setting sun

The setting sun looks oval in shape because the light from the sun is refracted at different rate when passes through the atmosphere.

Twinkling Star

The light of stars is refracted when passes through different region in the atmosphere. The angle of refraction varies a little from time to time. As a result, the stars look twinkling.

 

 

1. The value of  sini/sinr is called the refractive index of the medium and it gives you an indication of its light-bending ability.
   
2. In SPM, when we say “refractive index”, what we mean is the absolute refractive index of a substance. The absolute refractive index of a substance is the refractive index where light ray travels from vacuum (or air) into the substance.

Refractive Index and the Speed of light

or

( Note that the greater the refractive index of a medium, the lower is the speed of light. The more light is slowed, the more it is bent. )

 

1. The incident and refracted rays are on opposite sides of the normal at the point of incidence, and all three lie in the same plane.
2. The value of sini/sinr is constant for light passing from one given medium into another. This is known as Snell's law.

 

1. Light rays are bent when they pass at an angle in or out of materials such as glass and water. The effect is called refraction.
2. Light passing into an optically denser medium is bent towards the normal; light passing into an optically less dense medium is bent away from the normal.
3. Materials such as glass, water and paraffin are said to be optically denser than air.

 

Finding the Position and Size of the Image

1. Any two rays are sufficient to fix the position and size of the image. Look for the point where the rays cross after reflection from the mirror.
2. The interception of the two rays is the focus of the ray.

Example

The Ray Diagram and the Types of Image

The nature and size of the image formed by concave mirrors depends on the distance of the object from the mirror. This will be explained in the following sections.

 

Rules in Drawing Ray Diagram

Rule No. 1
The ray of light through C will be reflected back through C.

Rule No. 2
The ray of light parallel to the principal axis will be reflected through F.

Rule No. 3

The ray of light through F will be reflected parallel to the principal axis.

 

Curved Mirror

1. A curve is part of a circle. Therefore
   1. the centre of the circle will also be the centre of the curve and is called the centre of curvature, and
   2. the radius of the circle will be equal to the radius of the curve, called the curvature radius.

Important Terms

All rays parallel to the principle axis will focus at F

 

Steps to draw a ray diagram for an image in a plane mirror 

Step 1

( Draw the virtual image. Distance of object = Distance of image )

Step 2

( Draw 2 reflected rays, one from the image to the top of the eye and the other one from the image from the bottom of the eye. )

Step 3

( Draw the respective incident rays for the reflected rays you draw in step 2. )