Home › Archive for SPM Physics › Page 19

SPM Physics

2.10.3 Vectors in Equilibrium

Posted on by

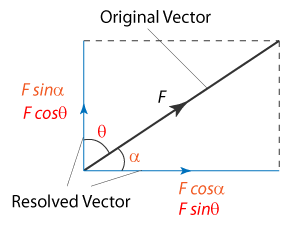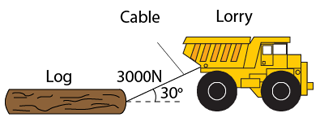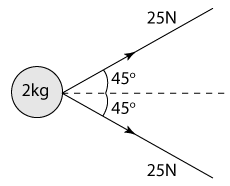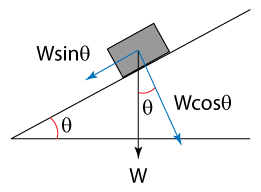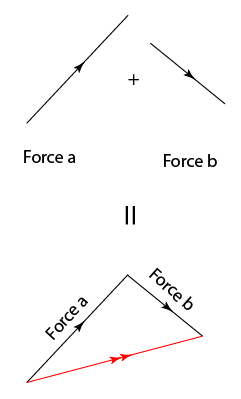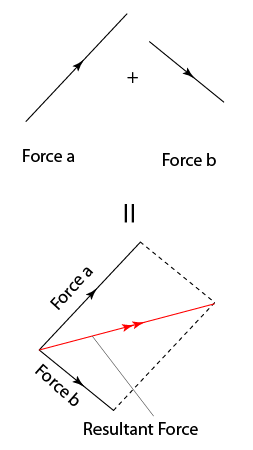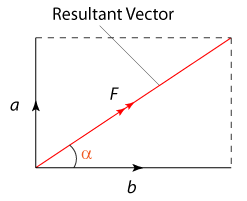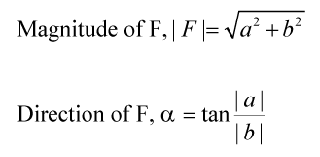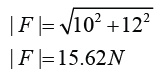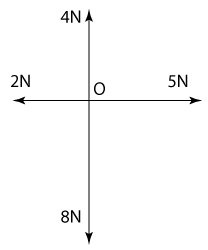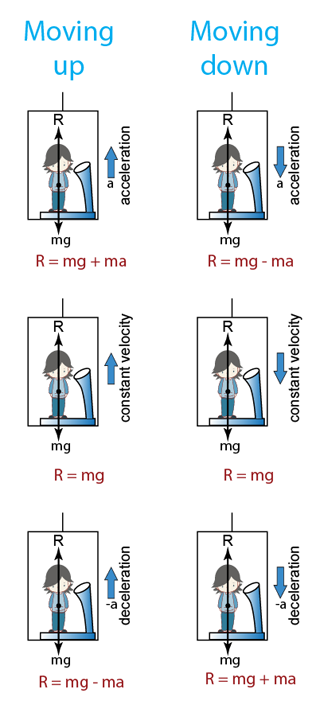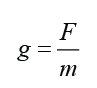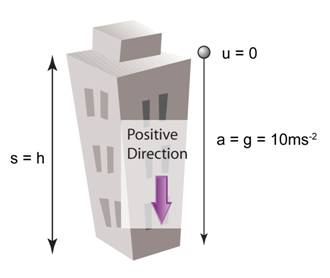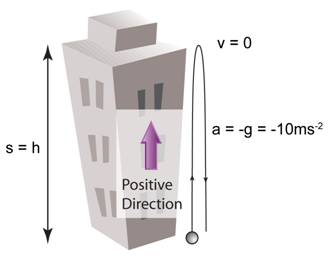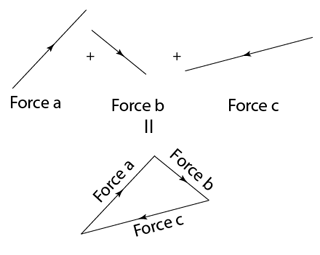
When 3 vectors are in equilibrium, the resultant vector = 0. After joining all the vectors tail to head, the head of the last vector will join to the tail of the first vector.

Forces in equilibrium

Forces are in equilibrium means the resultant force in all directions are zero.
When the forces acting on an object are balanced, they cancel each other out. The net force is zero.

Effect :
  • an object at rest is continuely at rest [ velocity = 0]
  • a moving object will move at constant velocity [ a = 0]

Example:

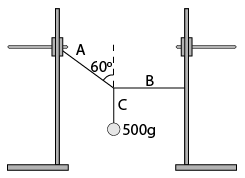
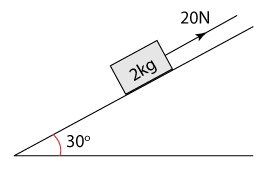
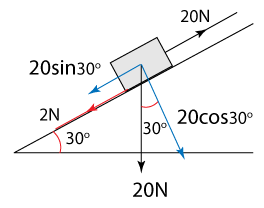
Diagram above shows a load of mass 500g is hung on a string C, which is tied to 2 other strings A and B. Find the tension of string A.

Answer:

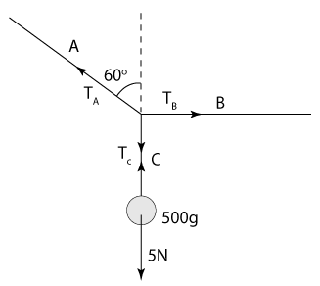
Tension of string C, TC = weight of the load = 5N
All forces in the system are in equilibrium, hence

Vertical component of tension A (TA) = TC
TAcos60o = TC
TA = TC/cos60o
TA = 5/cos60o = 10N

 

2.10.2 Vector Resolution

Posted on by


A vector can be resolve into 2 component which is perpendicular to each others.

Example:

Diagram above shows a lorry pulling a log with an iron cable. If the tension of the cable is 3000N and the friction between the log and the ground is 500N, find the horizontal force that acting on the log.

 Answer:
Horizontal component of the tension = 3000 cos30° =2598N
Friction = 500N

Resultant horizontal force = 2598N - 500N =2098N

Example:

Diagram above shows two forces of magnitude 25N are acting on an object of mass 2kg. Find the acceleration of object P, in ms-2.

 Answer:
Horizontal component of the forces = 25cos45° + 25cos45° = 35.36N

Vertical component of the forces = 25sin45° - 25sin45° = 0N

The acceleration of the object can be determined by the equation

F = ma
(35.36) = (2)a
a = 17.68 ms-2

Inclined Plane


Weight component along the plane = Wsinθ.
Weight component perpendicular to the plane = Wcosθ.

Example:

A block of mass 2 kg is pulling along a plane by a 20N force as shown in diagram above. Given that the fiction between block and the plane is 2N, find the magnitude of the resultant force parallel to the plane.
Answer:


First of all, let's examine all the forces or component of forces acting along the plane.

The force pulling the block, F = 20N
The frictional force Ffric = 2N
The weight component along the plane = 20sin30° = 10N

The resultant force along the plane = 20 - 2 - 10 = 8N

 

2.10.1 Vector and Scalar Quantity

Posted on by
  1. A scalar quantity is a quantity which can be fully described by magnitude only.
  2. A vector quantity is a quantity which is fully described by both magnitude and direction.

Vector Diagram

  1. The arrow shows the direction of the vector.
  2. The length representing the magnitude of the vector.

Equal Vector

Two vectors A and B may be defined to be equal if they have the same magnitude and point in the same direction.

Vector Addition - Triangle Method


Join the tail of the 2nd vector to the head of the 1st vector. Normally the resultant vector is marked with double arrow.

Vector Addition - Parallelogram Method


Join the tail of the 2nd vector to the tail of the 1st vector. Normally the resultant vector is marked with double arrow.

Addition of 2 Perpendicular Vectors


If 2 vectors (a and b) are perpendicular to each others, the magnitude and direction of the resultant vector can be determined by the following equation.


Example:
Two forces, P and Q of magnitude 10N and 12N are perpendicular to each others. What is the magnitude of the resultant force if P and Q are acting on an object?

 Answer:
Magnitude of the resultant force



Example:

Diagram above shows that four forces of magnitude 2N, 4N, 5N and 8N are acting on point O. All the forces are perpendicular to each others. What is the magnitude of the resulatant force that acts on point O?

 Answer:
The resultant force of the horizntal component = 5 - 2 = 3N to the right
The resultant force of the vertical component = 8 - 4 = 4N acting downward.

Therefore, the magtitude of these 2 force components,

 

2.9.3 Apparent Weight in a Lift

Posted on by

  1. When a man standing inside an elevator, there are two forces acting on him.
    1. His weight, (W) which acting downward.
    2. Normal reaction (R), acting in the opposite direction of weight.
  2. The reading of the balance is equal to the normal reaction (R).
  3. Figure below shows the formula to calculate the reading of the balance at different situation.



Example 1:
Subra is standing on a balance inside an elevator. If Subra’s mass is 63kg, find the reading of the balance when the lift,
  1. stationary
  2. moving upward with a constant velocity, 15 ms-1.,
  3. moving upward with a constant acceleration, 1 ms-2.
  4. moving downward with a constant acceleration, 2 ms-2.

Answer:
a.

W = mg
W = (63)(10) = 630N
b.

W = mg
W = (63)(10) = 630N

c.

R = mg + ma
R = (63)(10) + (63)(1)
R = 693N
d.

R = mg - ma
R = (63)(10) - (63)(2)
R = 630 - 126 = 504N


Example 2:
A 54kg boy is standing in an elevator. Find the force on the boy's feet when the elevator
  1. stands still
  2. moves downward at a constant velocity of 3 m/s
  3. decelerates downward with at 4.0 m/s2, 
  4. decelerates upward withat 2.0 m/s2.

Answer:
a.

W = mg
W = (54)(10) = 540N
b.

W = mg
W = (54)(10) = 540N
c.

R = mg + ma
R = (54)(10) + (54)(4) = 756N

d.

R = mg - ma
R = (54)(10) - (54)(2) = 432N

 

2.9.2 Weight and Mass

Posted on by

Mass

  1. Mass is defined as the quantity of matter. It is a base quantity and also a scalar quantity, which mean it has no direction.
  2. The SI unit of mass is kilogram (kg)

Weight

  1. The weight of an object is defined as the gravitational force acting on the object.
  2. The SI unit of weight is Newton (N)

Differences between Weight and Mass

Weight Mass
Depends on the gravitational field strengthIndependent from the gravitational field strength
Vector quantityScalar Quantity
Unit Newton (N)Unit: Kilogram (kg)

 

 

2.9.1 Gravitational Field

Posted on by

A gravitational field as a region in which an object experiences a force due to gravitational attraction.

Gravitational Field Strength

  1. The gravitational field strength at a point in the gravitational field is the gravitational force acting on a mass of 1 kg placed at that point.
  2. The unit of gravitational field strength is N/kg.
  3. The gravitational field strength is denoted by the symbol "g".
    4. g = Gravitational Field Strength
    F = Force acted on an object
    m = mass of the object.

Gravitational Acceleration

  1. The gravitational acceleration is the acceleration of an object due to the pull of the gravitational force. It has the unit of ms-2
  2. The symbol of gravitational acceleration is " g ".
  3. Gravitational acceleration does not depend on the mass of the moving object.
  4. The magnitude of gravitational acceleration is taken to be 10ms-2.

Important notes:

  1. Gravitational acceleration does not depend on the mass of the moving object.
  2. The magnitude of gravitational acceleration is taken to be 10ms-2.

Gravitational Field Strength vs. Gravitational Acceleration

  1. Both the gravitational field strength and gravitational acceleration have the symbol, g and the same value (10ms-2) on the surface of the earth.
  2. When considering a body falling freely, the g is the gravitational acceleration.
  3. When considering objects at rest, g is the Earth’s gravitational field strength acting on it.

Free Falling

  1. Free falling is a motion under force of gravity as the only force acting on the moving object.
  2. Practically, free falling can only take place in vacuum.

Case of Free Falling 1 - Falling from High Place

When an object is released from a high place,

  1. its initial velocity, u = 0.
  2. its acceleration is equal to the gravitational acceleration, g, which taken to be 10ms-2 in SPM.
  3. the displacement is the of the object when it reaches the ground is equal to the initial height of the object, h.

Case of Free Falling 2 - Launching Object Upward

If an object is launched up vertically,

  1. the acceleration = -g (-10ms-2)
  2. the velocity become zero when the object reaches the highest point.
  3. the displacement of the object at highest point is equal to the vertical height of object, h
  4. the time taken for the object to move to the maximum height = the time taken for the object to fall from the maximum point to its initial position.

 

2.8.1 Safety Features of Vehicle

Posted on by

Crumble Zone

 


The crumple zone increases the reaction time of collision during an accident.
This causes the impulsive force to be reduced and hence reduces the risk of injuries.

Seat Belt


Prevent the driver and passengers from being flung forward or thrown out of the car during an emergency break.

Airbag


The inflated airbag during an accident acts as a cushion to lessen the impact when the driver flings forward hitting the steering wheel or dashboard.

Head Rest


Reduce neck injury when driver and passengers are thrown backwards when the car is banged from backward.

Windscreen


Shatter-proof glass is used so that it will not break into small pieces when broken. This may reduce injuries caused by scattered glass.

Padded Dashboard


Cover with soft material. This may increases the reaction time and hence reduce the impulsive force when passenger knocking on it in accident.

Collapsible Steering Columns


The steering will swing away from driver’s chest during collision. This may reduce the impulsive force acting on the driver.

Anti-lock Braking System (ABS)


Prevent the wheels from locking when brake applied suddenly by adjusting the pressure of the brake fluid. This can prevents the car from skidding.

Bumper


Made of elastic material so that it can increases the reaction time and hence reduces the impulsive force caused by collision.

Passenger Safety Cell


The body of the car is made from strong, rigid stell cage.
This may prevent the car from collapsing on the passengers during a car crash.

 

2.7.2 Impulse

Posted on by
Impulse is defined as the product of the force (F) acting on an object and the time of action (t). 
  1. Impulse is the product between the force, F with the time of impact, t.
  2. Impulse is also defined as the change in momentum.
  3. Impulse is a vector quantity.
  4. An impulse will cause velocity change of an object.

Formula of impulse

Impulse is the product of force and time.

Impulse = F x t

Impulse = change of momentum

Impulve = mv - mu


Example:
A billiard ball weighing 0.25 kg is in a stationary state on a smooth billiard table. The ball is then given an impulse as much as 3.0Ns horizontally. What is the velocity of the ball after impact?

 Answer:
Mass of the billiard ball, m = 0.25kg
Initial velocity of the billiard ball, u = 0
Impulse = 3.0Ns
Final velocity, v = ?

Impulse = momentum change = mv - mu
(3.0) = (0.25)v - (0.25)(0)
3 = 0.25v
v = 3/0.25 = 12 m/s

 

2.7.1 Impulsive Force

Posted on by
  1. Impulsive force is defined as the rate of change of momentum in a reaction. Mathematically, we write this as
  2. It is a force which acts on an object for a very short interval during a collision or explosion.

Example:
A car of mass 1000kg is traveling with a velocity of 25 m/s. The car hits a street lamp and is stopped in0.05 seconds. What is the impulsive force acting on the car during the crash?

 Answer:
m = 1000kg
u = 24 m/s
v = 0
t = 0.05s


Effects of impulse vs Force

  • A force determines the acceleration (rate of velocity change) of an object. A greater force produces a higher acceleration.
  • An impulse determines the velocity change of an object. A greater impulse yield a higher velocity change.
Examples Involving Impulsive Force
  1. Playing football
  2. Playing badminton
  3. Playing tennis
  4. Playing golf
  5. Playing baseball

Long Jump


  1. The long jump pit is filled with sand to increase the reaction time when atlete land on it.
  2. This is to reduce the impulsive force acts on the leg of the atlete because impulsive force is inversely proportional to the reaction time.

High Jump


(This image is licenced under the GNU Free Document Licence. The original file is from the Wikipedia.org.)

  • During a high jump, a high jumper will land on a thick, soft mattress after the jump.
  • This is to increase the reaction time and hence reduces the impulsive force acting on the high jumper.

Jump off From a High Place

A jumper bends his/her leg during landing. This is to increase the reaction time and hence reduce the impact of impulsive force acting on the leg of the jumper.

 

2.6.2 Force, Mass and Acceleration

Posted on by

Newton's Second Law

The rate of change of momentum of a body is directly proportional to the resultant force acting on the body and is in the same direction.

Implication:

When there is resultant force acting on an object, the object will accelerate (moving faster, moving slower or change direction).


Formula of Force


From Newton's Second Law, we can derived the equation
(IMPORTANT: F Must be the net force)

Summary of Newton's 1st Law and 2nd Law

Newton's First Law:
When there is no net force acting on an object, the object is either stationary or move with constant speed in a straight line.

Newton's Second Law:
When there is a net force acting on an object, the object will accelerate.

Example:
A box of mass 150kg is placed on a horizontal floor with a smooth surface; find the acceleration of the box when a 300N force is acting on the box horizontally.

Answer:
F = ma
(300) = (150)a
a = 2 ms-2

Example:
A object of mass 50kg is placed on a horizontal floor with a smooth surface. If the velocity of the object changes from stationary to 25.0 m/s in 5 seconds when is acted by a force, find the magnitude of the force that is acting?

Answer:
We know that we can find the magnitude of a force by using the formula F = ma. The mass m is already given in the question, but the acceleration is not give directly.

We can determine the acceleration from the formula

From the formula
F = ma = (50)(5) = 250N

The force acting on the box is 250N.