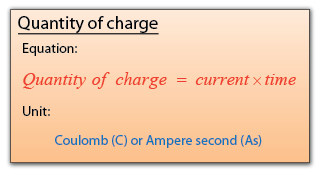
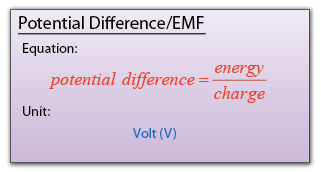
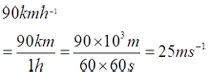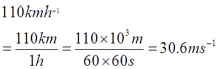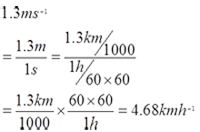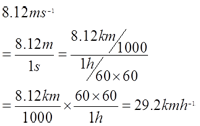Ruler

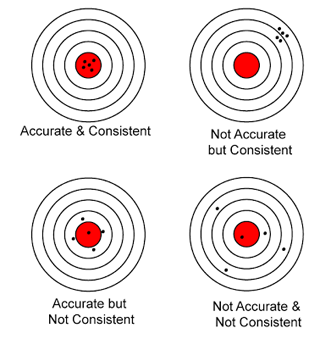
A metre rule has sensitivity or accuracy accuracy of 1mm. Precaution to be taken when using ruler
- Make sure that the object is in contact with the ruler.
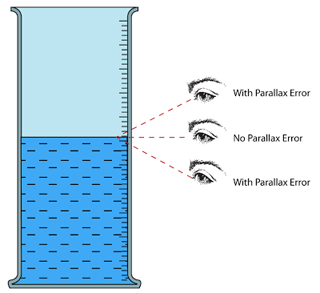
- Avoid parallax error.
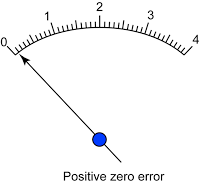
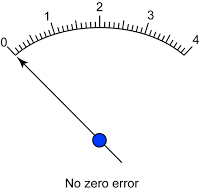
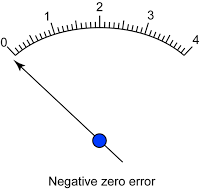
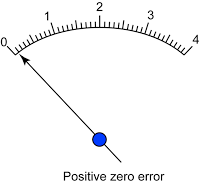
- Avoid zero error and end error.
Thermometer

- Thermometers of range -10°C - 110°C with accuracy 1°C.
- Thermometers of range 0°C - 360°C with accuracy 2°C.
- Make sure that the temperature measured does not exceed the measuring range.
- When measuring temperature of liquid.
- immerse the bulb fully in the liquid
- stir the liquid so that the temperature in the liquid is uniform
- do not stir the liquid vigorously to avoid breaking the thermometer
Stopwatch

(The image is licienced under GDFL. The source file can be obtained at wikipedia.org.)
- analogue stopwatches of sensitivity 0.1s or 0.2s
- digital stopwatches of sensitivity 0.01s.