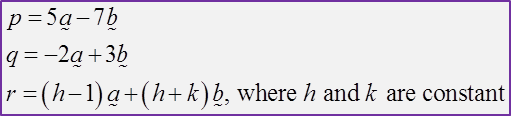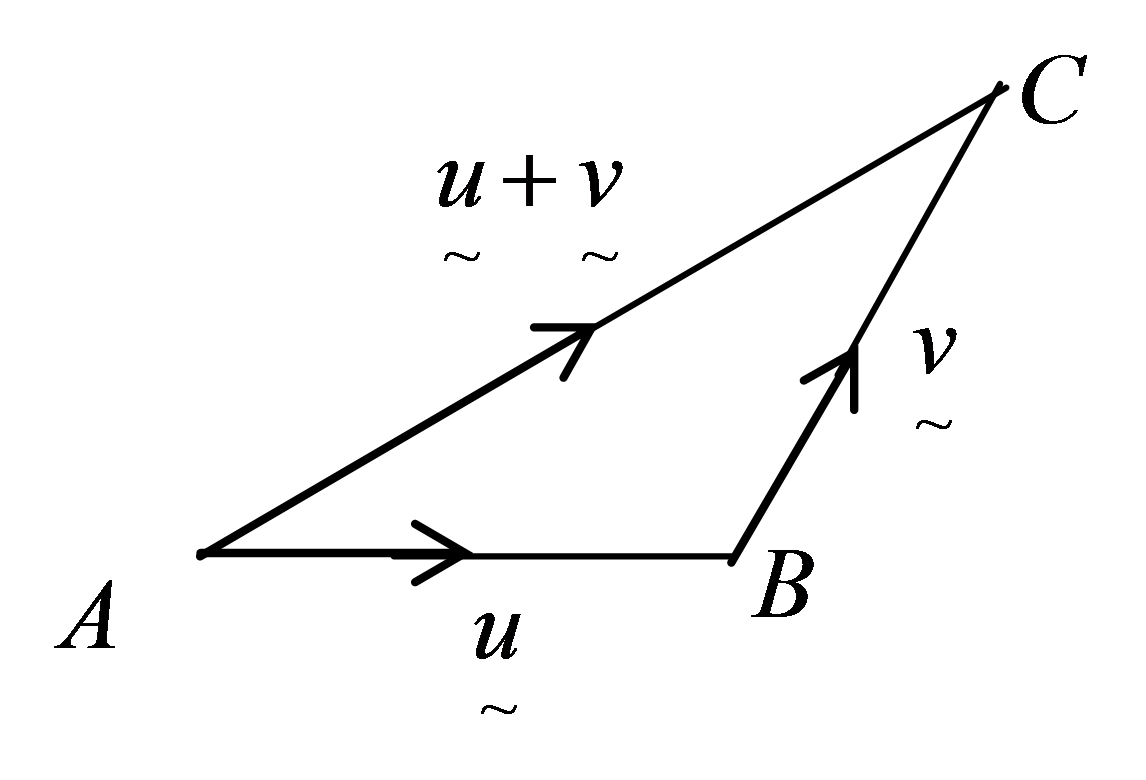Short Question 6 & 7
Question 6:
The points P, Q and R are collinear. It is given that and , where k is a constant. Find
(a) the value of k,
(b) the ratio of PQ : QR.
Solution:
(a)
(b)
The points P, Q and R are collinear. It is given that and , where k is a constant. Find
(a) the value of k,
(b) the ratio of PQ : QR.
Solution:
(a)
(b)
Question 7:
Given that and , find the values of m if the vector is parallel to the vector .
Solution:
Given that and , find the values of m if the vector is parallel to the vector .
Solution:
4.2 Multiplication of Vector by a Scalar and the Parallel Condition of Two Vectors
4.2 Multiplication of Vector by a Scalar and the Parallel Condition of Two Vectors
1. When a vector
is multiplied by a scalar k, the product is
. Its magnitude is k times the magnitude of the vector
.
2. The vector is parallel to the vector if and only if , where k is a constant.
3. If the vectors
and
are not parallel and
, then h = 0 and k = 0.
Example 1:
If vectors
are not parallel and
, find the value of k and of h.
Solution:
The vectors
are not parallel, so
k – 7 = 0 → k = 7
5 + h = 0 → h = –5
4.3 Addition and Subtraction of Vectors
4.3.2 Subtraction of Vectors
The subtraction of the vector
from the vector
is written as
. This operation can be considered as the addition of the vector
with the negative vector of
. Therefore
Example 1:
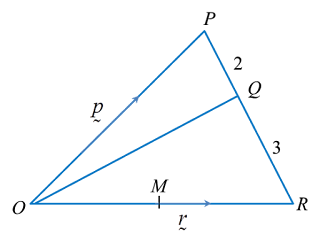
In the diagram above, vector and Q divides PR in the ratio of 2 : 3. Find the following vectors in terms of
Solution:
(a)
(b)
(c)
Long Question 5
Question 5:
Diagram below shows a triangle KLM.
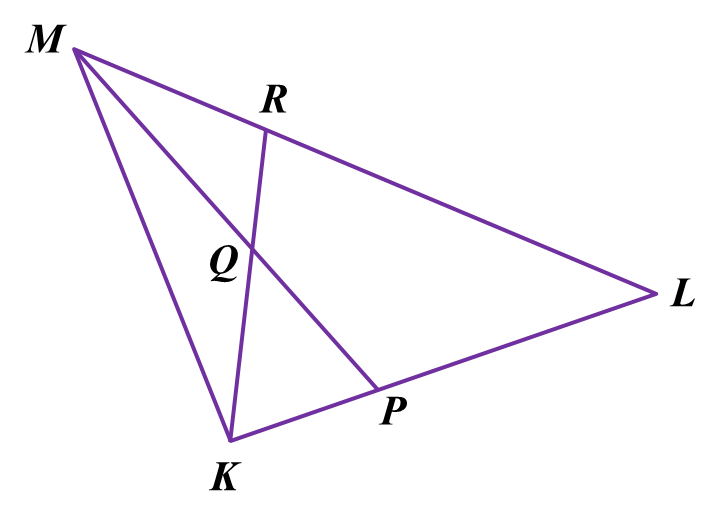
Solution:
(a)(i)
(a)(ii)
(b)
(c)
Diagram below shows a triangle KLM.

Solution:
(a)(i)
(a)(ii)
(b)
(c)
Short Question 4 & 5
Long Question 2
Question 2:
Given that and , find
(a) the coordinates of A,
(b) the unit vector in the direction of .
(c) the value of m if CD is parallel to AB .
Solution:
(a)
(b)
(c)
Given that and , find
(a) the coordinates of A,
(b) the unit vector in the direction of .
(c) the value of m if CD is parallel to AB .
Solution:
(a)
(b)
(c)
Vectors In Cartesian Plane
(A) Vectors in Cartesian Coordinates
1. A unit vector is a vector whose magnitude is one unit.
2. A unit vector that is parallel to the x-axis is denoted by
while a unit vector that is parallel to the y-axis is denoted by
.
3. The unit vector can be expressed in columnar form as below:
4. The magnitudes of the unit vectors are
5. The magnitude of the vector
can be calculated using the Pythagoras’ Theorem.
(B) Unit Vector in the Direction of a Vector
Example 1:
Solution:
Example 1:
If
and
, find the values of k. Determine the unit vector in the direction of
for each value of k.
Solution:
Example 2:
It is given that
(a) Find
(b) Hence, find the unit vector in the direction of
Solution:
(a)
(b)
Short Question 1 – 3
Question 1:
Given that O (0, 0), A (–3, 4) and B (–9, 12), find in terms of the unit vectors, and
Solution:
(a)
(b)
Given that O (0, 0), A (–3, 4) and B (–9, 12), find in terms of the unit vectors, and
(a)
(b) the unit vector in the direction of
Solution:
(a)
(b)
Question 2:
Given that A (–3, 2), B (4, 6) and C (m, n), find the value of m and of n such that
Solution:
Given that A (–3, 2), B (4, 6) and C (m, n), find the value of m and of n such that
Solution:
4.3.1 Addition of Vectors
4.3 Addition and Subtraction of Vectors
4.3.1 Addition of Vectors
1. The addition of two vectors,
, can be written as
. The result of this addition is a vector which is called the resultant vector.
2. When two vectors with the same direction is added up, the resultant vector has
2. When two vectors with the same direction is added up, the resultant vector has
(a) the same direction with both the vectors.
(b) a magnitude equal to the sum of the magnitudes of both the vectors.
Addition of Non-parallel Vectors
1. Addition of two non-parallel vectors,
, can be shown by using two laws.
(a) Triangle Law of Addition
The resultant vector
is represented by the third side AC.
(b) Parallelogram Law of Addition
(b) Parallelogram Law of Addition
The resultant vector
is represented by the third side AC.