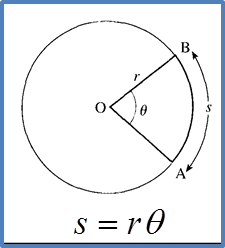
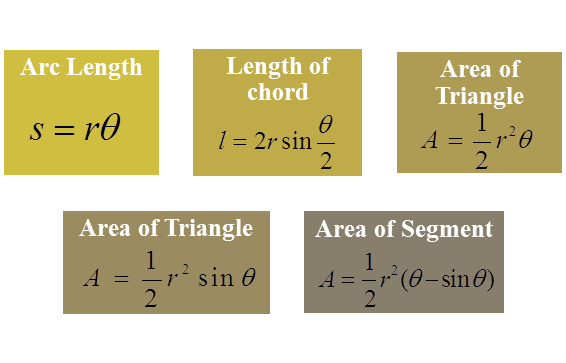Method 2 (Differentiate Directly)
Example:
Given that y=x22x+1, find dydx
Solution:
y=x22x+1dydx=(2x+1)(2x)−x2(2)(2x+1)2 =4x2+2x−2x2(2x+1)2=2x2+2x(2x+1)2
Example:
Given that y=x22x+1, find dydx
Solution:
y=x22x+1dydx=(2x+1)(2x)−x2(2)(2x+1)2 =4x2+2x−2x2(2x+1)2=2x2+2x(2x+1)2
Practice 1:
Given that y=4x3(5x+1)3, find dydx
Solution:
y=4x3(5x+1)3dydx=(5x+1)3(12x2)−4x3.3(5x+1)2.5[(5x+1)3]2 =(5x+1)3(12x2)−60x3(5x+1)2(5x+1)6 =(12x2)(5x+1)2[(5x+1)−5x](5x+1)6 =(12x2)(5x+1)2(1)(5x+1)6 =12x2(5x+1)4
Given that y=4x3(5x+1)3, find dydx
Solution:
y=4x3(5x+1)3dydx=(5x+1)3(12x2)−4x3.3(5x+1)2.5[(5x+1)3]2 =(5x+1)3(12x2)−60x3(5x+1)2(5x+1)6 =(12x2)(5x+1)2[(5x+1)−5x](5x+1)6 =(12x2)(5x+1)2(1)(5x+1)6 =12x2(5x+1)4

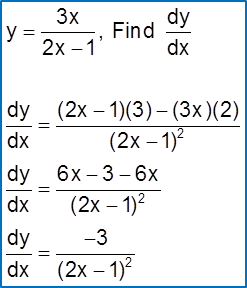

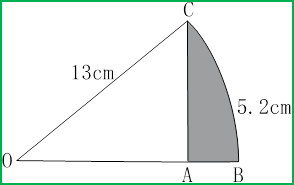
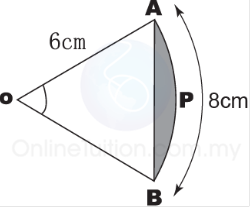
 It is given that OP = 17 cm and PQ = 8.8 cm.
It is given that OP = 17 cm and PQ = 8.8 cm. [Use π = 3.142]
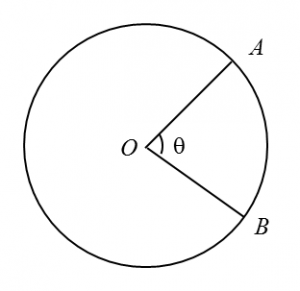
[Use π = 3.142]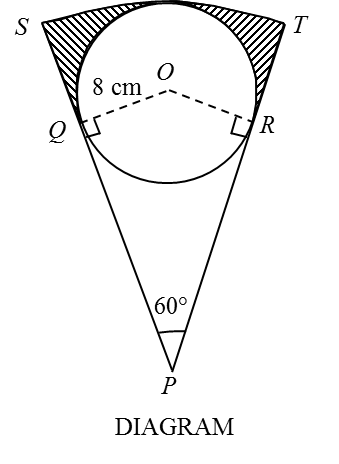
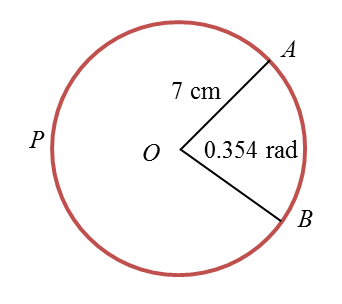
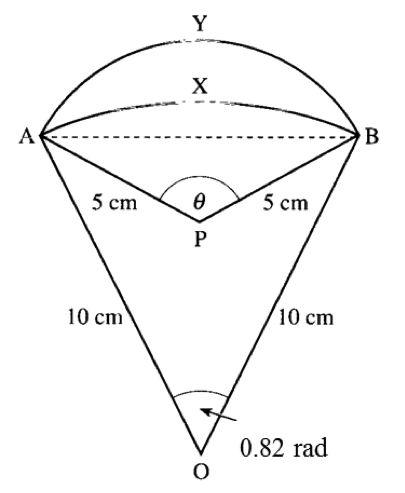
 Given that OQ = 8 cm, PY = 3 cm ,
Given that OQ = 8 cm, PY = 3 cm ,