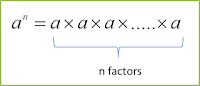Indices and Laws of Indices (Part 1)

Positive Integral Indices

When a real number a is multiplied by itself n times, the result is the nth power of a.

Example: 5×5×5×5 = 5⁴(5 to the power of 4)

In general, if a is any real number and n is a positive integer, then

The integer n is called the index or exponent and a is the base.

5.1 Indices and Laws of Indices (Part 1)

(A) Zero Indices

The zero index of any number is equal to one.

a ⁰ = 1, where a ≠ 0

Example 1:

Find the value of the followings:

(a) 250⁰

(b) 0.513⁰

\begin{array}{l} (c) {(\frac{2}{7})}^{0} \\ (d) {(- 11 \frac{1}{25})}^{0} \end{array}

Solution:

(a) 250⁰ = 1

(b) 0.513⁰ = 1

\begin{array}{l} (c) {(\frac{2}{7})}^{0} = 1 \\ (d) {(- 11 \frac{1}{25})}^{0} = 1 \end{array}

(B) Negative Integral Indices

\begin{array}{l} a^{- n} is a reciprocal of a^{n} . \\ a^{- n} = \frac{1}{a^{n}} \end{array}

Example 2:

Find the value of the followings:

(a) 102 ^-1

(b)–6 ^-3

\begin{array}{l} (c) {(\frac{1}{3})}^{- 4} \\ (d) {(\frac{2}{5})}^{- 2} \\ (e) - {(\frac{2}{5})}^{- 4} \end{array}

Solution:

\begin{array}{l} (a) 102^{- 1} = \frac{1}{102} \\ (b) - 6^{- 3} = \frac{1}{- 6^{3}} = - \frac{1}{216} \\ (c) {(\frac{1}{3})}^{- 4} = {(3)}^{4} = 81 \\ (d) {(\frac{2}{5})}^{- 2} = {(\frac{5}{2})}^{2} = \frac{25}{4} \\ (e) {(- \frac{2}{5})}^{- 4} = {(- \frac{5}{2})}^{4} = \frac{625}{16} \end{array}