SPM Practice Question 10 – 12

Question 10:
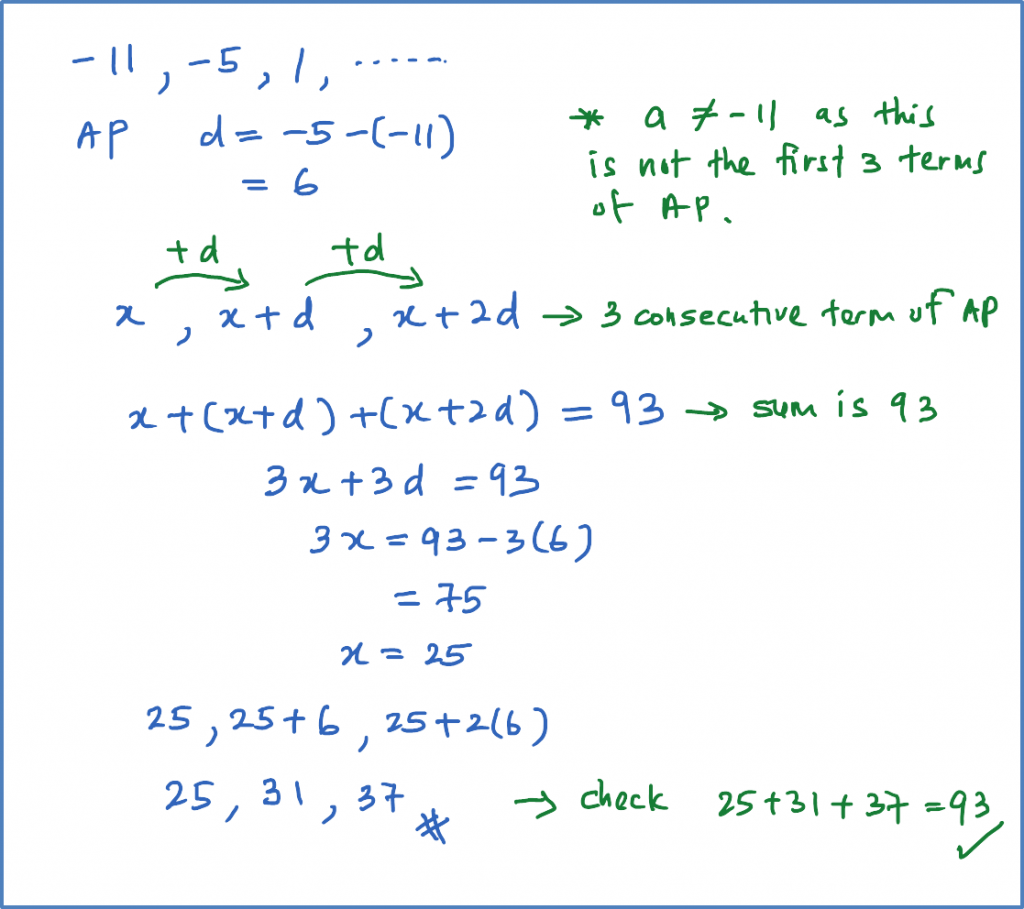
The sequence –11, –5, 1,… is an arithmetic progression. State the three consecutive terms of this arithmetic progression where the sum of these three terms is 93.

Solution:

Question 11:
An arithmetic series, with the first term 12 and common difference d, consists of 23 terms. Given that the sum of the last 3 terms is 5 times the sum of the first 3 terms, find
(a) the value of d,
(b) the sum of the first 19 terms.

Solution:

Question 12:
The sum of n terms of an arithmetic progression is given by the formula $S_n=\frac{n}{2}(5-3n)$ . Find
(a) the first term,
(b) the common difference,
(c) the tenth term.

Solution: