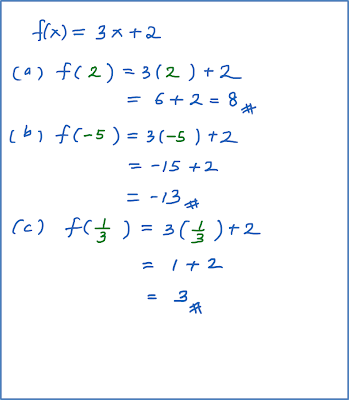Functions
Example 1
If f : x → x + 5 and g : x → x2 +2x + 3, find
- the value of gf (2),
- the value of fg (2 ),
- the composite functions fg,
- the composite functions gf,
- the composite functions g2 ,
- the composite functions f2.

correction for part (c)
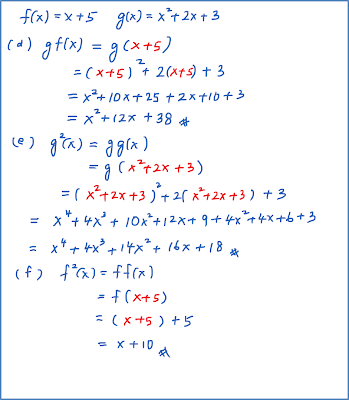
1.3a Composite Function
Composite Function
If function
,
and function ,
hence, composite function
and function ,
hence, composite function

Example:
If, and , find
Answer:
If, and , find
Answer:
Example 4 and 5
Example 2 and 3
Domain, Range, Objects, Images and Absolute Value Functions
(B) Domain, Range, Objects and Images of a Function
Example:

The arrow diagram above represents the function f : x → 2x2 – 5. State
(a) the domain,
(b) the range,
(c) the image of –2,
(d) the objects of
(i) –3,
(ii) –5.
Solution:
(a) Domain = {–2, –1, 0, 1, 2}.
(b) Range = {–5, –3, 3}.
(c) The image of –2 is 3.
(d) (i) The objects of –3 are 1 and –1.
(d) (ii) The objects of –5 is 0.
Example:

The arrow diagram above represents the function f : x → 2x2 – 5. State
(a) the domain,
(b) the range,
(c) the image of –2,
(d) the objects of
(i) –3,
(ii) –5.
Solution:
(a) Domain = {–2, –1, 0, 1, 2}.
(b) Range = {–5, –3, 3}.
(c) The image of –2 is 3.
(d) (i) The objects of –3 are 1 and –1.
(d) (ii) The objects of –5 is 0.
(C) Absolute Value Functions
1. Symbol | | is read as ‘the modulus’ of a number. In general, the modulus of x, that is | x |, is defined as
2. In other words, modulus of a number always positive.
3. The absolute value function | f(x) | is defined by
Solution:
(a)
Given f (x) = |x + 2|
Image of –4 is f(–4) = | –4 + 2| = | –2| = 2
Image of –3 is f(–3) = | –3 + 2| = | –1| = 1
Image of 0 is f(0) = | 0 + 2| = | 2 | = 2
Image of 2 is f(2) = | 2 + 2| = | 4 | = 4
(b)
From (a),
f(–4) = 2
f(–3) = 1
f(0) = 2
f(2) = 4
Determine the point where the graph touches the x-axis.
At x-axis, f (x) = 0
|x + 2| = 0
x + 2 = 0
x = –2

Therefore, range of values of f (x) is 0 ≤ f (x) ≤ 4.
1. Symbol | | is read as ‘the modulus’ of a number. In general, the modulus of x, that is | x |, is defined as
2. In other words, modulus of a number always positive.
3. The absolute value function | f(x) | is defined by
Example:
Given function f : x → |x + 2|.
(a) Find the image of –4, –3, 0 and 2.
(b) Sketch the graph of f (x) for the domain –4 ≤ x ≤ 2.
Hence, state the range of values of f (x) based on the given domain.
Solution:
(a)
Given f (x) = |x + 2|
Image of –4 is f(–4) = | –4 + 2| = | –2| = 2
Image of –3 is f(–3) = | –3 + 2| = | –1| = 1
Image of 0 is f(0) = | 0 + 2| = | 2 | = 2
Image of 2 is f(2) = | 2 + 2| = | 4 | = 4
(b)
From (a),
f(–4) = 2
f(–3) = 1
f(0) = 2
f(2) = 4
Determine the point where the graph touches the x-axis.
At x-axis, f (x) = 0
|x + 2| = 0
x + 2 = 0
x = –2

Therefore, range of values of f (x) is 0 ≤ f (x) ≤ 4.
Notation of Function
As shown in figure above, for a function
, each element x in the domain X has a unique image y in the codomain Y.
The function can be written as:
- For , we say y is a function of x.
- f(x) is also called the value of the function f at x.
- f(x) is read as "f of x".
Example:
Given the function , find the value of
a.
b.
c.
Answer:
(a)
(b)
(c)
Given the function , find the value of
a.
b.
c.
Answer:
(a)
(b)
(c)
1.2 Function – An Introduction

- A function is a relation in which every element in the domain has a unique image (exactly one) in the codomain.
- One-to-one relation and many-to-one relation are examples of a special kind of relation which we call function.

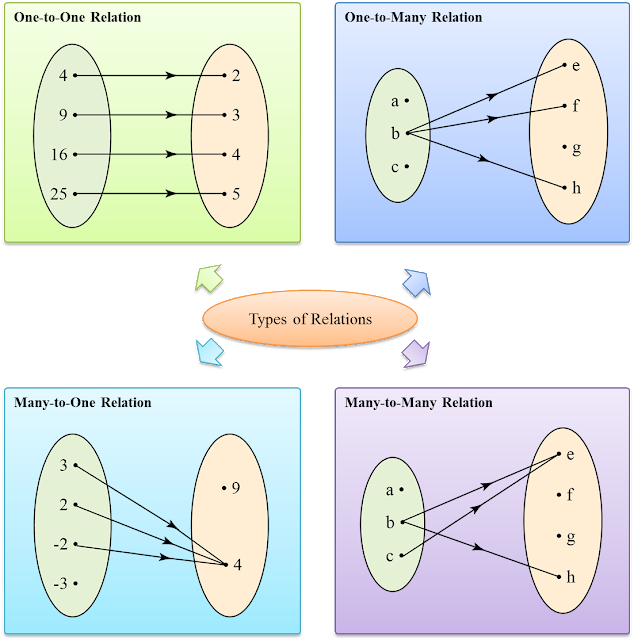
1.1.3 Types of Relation
- Relation can be classified into 4 types:
- One-to-one relation
- Many-to-one relation
- One-to-many relation
- Many-to-many relation
1.1b Domain and Codomain
[adinserter block="3"]
Domain and Codomain
- In the relation between one set and another, the first set is known as the domain and the second set is known as the codomain.
- Elements in the domain is called objects, whereas elements in the codomain mapped to the objects is called the image.
- Elements in the codomain not mapped to the objects are not the image.
- All images in codomain can be written as a set known as range.
Example:
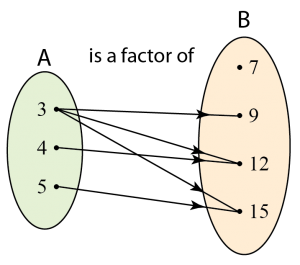
Domain = {3, 4, 5}
Codomain = {7, 9, 12, 15}
Range = {9, 12, 15} [Note: 7 is not an image because it is not mapped to any object.]
3 is the object of 9, 12 and 15.
4 is the object of 12.
5 is the object of 15.
9, 12 and 15 are the images of 3.
12 is the image of 4.
15 is the image of 5.

Domain = {3, 4, 5}
Codomain = {7, 9, 12, 15}
Range = {9, 12, 15} [Note: 7 is not an image because it is not mapped to any object.]
3 is the object of 9, 12 and 15.
4 is the object of 12.
5 is the object of 15.
9, 12 and 15 are the images of 3.
12 is the image of 4.
15 is the image of 5.