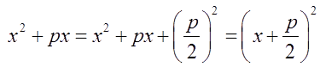SPM Add Maths
Finding the Sum of Roots (SoR) and Product of Roots (PoR)
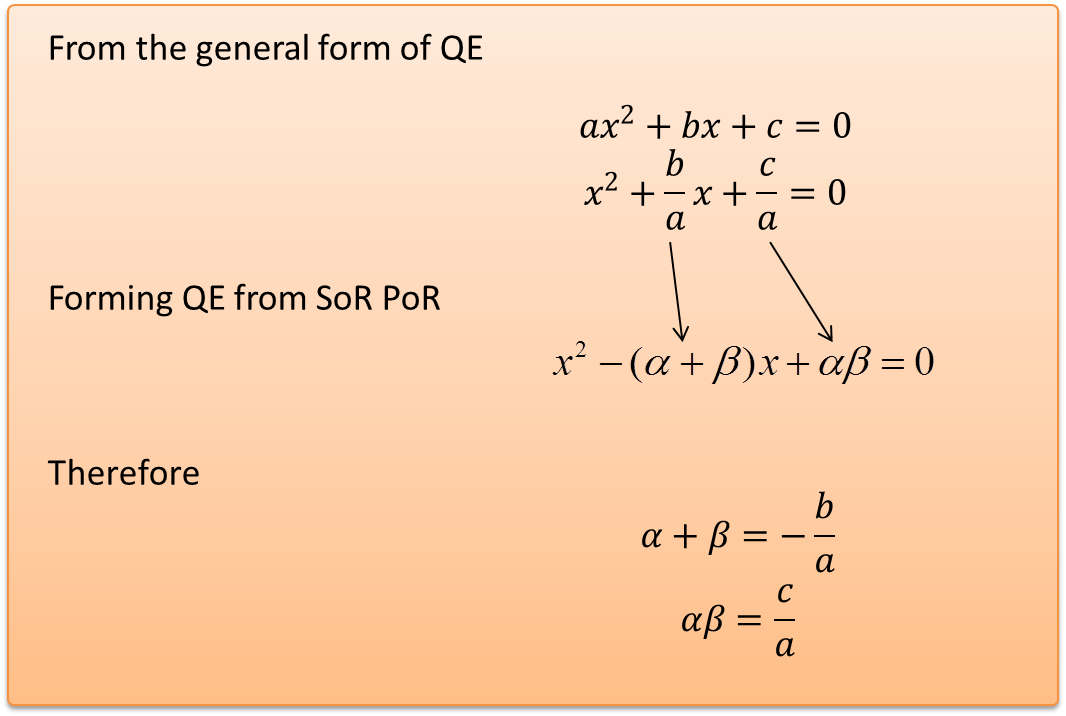
2.6 Finding the Sum of Roots (SoR) and Product of Roots (PoR)

Example
Find the sums and products of the roots of the following equations.
a. x2+7x−3=0x2+7x−3=0
b. x(x−1)=5(1−x)x(x−1)=5(1−x)
Answer:
(a)
x2+7x−3=0a=1, b=7, c=−3Sum of Rootsα+β=−ba=−71=−7Product of Rootsαβ=ca=−31=−3x2+7x−3=0a=1, b=7, c=−3Sum of Rootsα+β=−ba=−71=−7Product of Rootsαβ=ca=−31=−3
(b)
x(x−1)=5(1−x)x2−x=5−5xx2−x+5x−5=0x2+4x−5=0a=1, b=4, c=−5Sum of Rootsα+β=−ba=−41=−4Product of Rootsαβ=ca=−51−5x(x−1)=5(1−x)x2−x=5−5xx2−x+5x−5=0x2+4x−5=0a=1, b=4, c=−5Sum of Rootsα+β=−ba=−41=−4Product of Rootsαβ=ca=−51−5
Find the sums and products of the roots of the following equations.
a. x2+7x−3=0x2+7x−3=0
b. x(x−1)=5(1−x)x(x−1)=5(1−x)
Answer:
(a)
x2+7x−3=0a=1, b=7, c=−3Sum of Rootsα+β=−ba=−71=−7Product of Rootsαβ=ca=−31=−3x2+7x−3=0a=1, b=7, c=−3Sum of Rootsα+β=−ba=−71=−7Product of Rootsαβ=ca=−31=−3
(b)
x(x−1)=5(1−x)x2−x=5−5xx2−x+5x−5=0x2+4x−5=0a=1, b=4, c=−5Sum of Rootsα+β=−ba=−41=−4Product of Rootsαβ=ca=−51−5x(x−1)=5(1−x)x2−x=5−5xx2−x+5x−5=0x2+4x−5=0a=1, b=4, c=−5Sum of Rootsα+β=−ba=−41=−4Product of Rootsαβ=ca=−51−5
2.3a Forming Quadratic Equations from Given Roots
2.2.2c Solving Quadratic Equations – Quadratic Formula
The quadratic equation
ax2+bx+c=0ax2+bx+c=0
can be solved by using the quadratic formula
−b±√b2−4ac2a−b±√b2−4ac2a
Example
Use the quadratic formula to find the solutions of the following equations.
a. x2+5x−24=0x2+5x−24=0
b. x(x+4)=10x(x+4)=10
Answer
(a)
For the equation x2+5x−24=0x2+5x−24=0
(b)
x(x+ 4) = 10x2+4x−10=0a=1, b=4, c=−10x=−b±√b2−4ac2ax=−(4)±√(4)2−4(1)(−10)2(1)x=−4±√562x=1.742 or x=−5.742
−b±√b2−4ac2a−b±√b2−4ac2a
Example
Use the quadratic formula to find the solutions of the following equations.
a. x2+5x−24=0x2+5x−24=0
b. x(x+4)=10x(x+4)=10
Answer
(a)
For the equation x2+5x−24=0x2+5x−24=0
a = 1, b = 5, c = -24
x=−b±√b2−4ac2ax=−(5)±√(5)2−4(1)(−24)2(1)x=−5±√1212x=−8 or x=3x=−b±√b2−4ac2ax=−(5)±√(5)2−4(1)(−24)2(1)x=−5±√1212x=−8 or x=3
(b)
x(x+ 4) = 10x2+4x−10=0a=1, b=4, c=−10x=−b±√b2−4ac2ax=−(4)±√(4)2−4(1)(−10)2(1)x=−4±√562x=1.742 or x=−5.742
2.2b Solving Quadratic Equations – Completing the Square (Examples)
2.4.2a Solving Quadratic Equations – Completing the Square (Examples)
(A) Steps to solve quadratic equation using completing the square
• make sure that the coefficient of x is 1.
• Rewrite the equation ax2 + bx + c = 0 in the form ax2 + bx = –c.
• Add (coefficient of x2)2 to both side of the equation.
• make sure that the coefficient of x is 1.
• Rewrite the equation ax2 + bx + c = 0 in the form ax2 + bx = –c.
• Add (coefficient of x2)2 to both side of the equation.
Example:
Solve the following quadratic equations by completing the square.
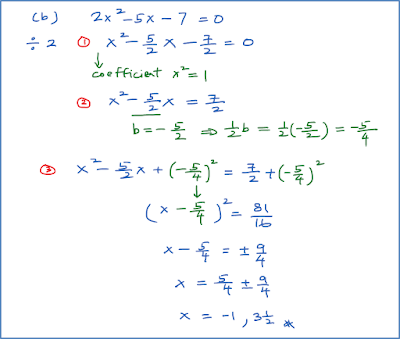
(a) x2 – 6x – 3 = 0
(a) x2 – 6x – 3 = 0
(b) 2x2 – 5x – 7 = 0
(c) x2 + 1 = 10x/3
Solution:
2.2b Solving Quadratic Equations – Completing the Square
2.4.2 Solving Quadratic Equations – Completing the Square
(A) The Perfect Square
(A) The Perfect Square
1. The expression x2 + 2x + 1 can be written in the form (x + 1)2, it is called a “perfect square”.
2. If the algebraic expression on the left hand side of the quadratic equation is a perfect, the roots can be easily obtained by finding the square roots.
2. If the algebraic expression on the left hand side of the quadratic equation is a perfect, the roots can be easily obtained by finding the square roots.
Example:
Solve each of the following quadratic equation
Solve each of the following quadratic equation
(a) (x + 1)2 = 25
(b) x2 − 8x + 16 = 49
Solution:
(a)
(x + 1)2 = 25
(x + 1)2 = ±√25
x = −1 ± 5
x = 5 or x = −6
(b)
x2 − 8x + 16 = 49
(x − 4)2 = 49
(x − 4) = ±√49
x = 4 ± 7
x = 11 or x = −3
(B) Solving Quadratic Equation by Completing the Square
1. To solve quadratic equation, we make the left hand side of the equation a perfect square.
2. To make any quadratic expression x2 + px into a perfect square, we add the term (p/2)2 to the expression and this will make
3. The following shows the steps to solve the equation by using completing the square method for quadratic equation ax2+ bx = – c.
(a) Rewrite the equation in the form ax2 + bx = – c.
(b) If the coefficient a ≠ 1, reduce it to 1 (by dividing).
(c) Add (p/2)2 to both sides of the equation.
(d) Write the expression on the left hand side as a perfect square.
(e) Solve the equation.
Solving Quadratic Equations
Solving Quadratic Equations
To solve a quadratic equation means to find all the roots of the quadratic equations.Example:
Find the roots of the quadratic equations
a. x2=9
b. 2x2−98=0
Answer:
(a)
x2=9x=±√9x=±3
(b)
2x2−98=02x2=98x2=982=49x=±√49=±7
Roots of Quadratic Equation – Example
General Form of Quadratic Equation
General Form of Quadratic Equation
General form of a quadratic equation is
ax2+bx+c=0
where a, b, and c are constants and a≠0.
*Note that the highest power of an unknown of a quadratic equation is 2.
General form of a quadratic equation is
ax2+bx+c=0
where a, b, and c are constants and a≠0.
*Note that the highest power of an unknown of a quadratic equation is 2.
Quadratic Equations (Introduction)
What is a Quadratic Equation?
- A quadratic equation is a polynomial equation of the second degree.
- A quadratic equation has only one variable
- The highest power of the variable is 2.
The followings are some examples of quadratic equations
- 2x2+3x+4=0
- t2=25
- y(6y−3)=5
- a−3y+2y2=2 where a is a constant.
Example of Non Quadratic Equation
- 2x+1=0 , (Reason: The highest power of x ≠ 2.)
- 2x3+1=x , (Reason: The highest power of x ≠ 2.)
- t2+5t=3 , (Reason: The present of the term 5t .)