Home › SPM Add Maths › Archive for Quadratic Equations

Quadratic Equations

Quadratic Equations Long Questions (Question 11 – 13)

Posted on by
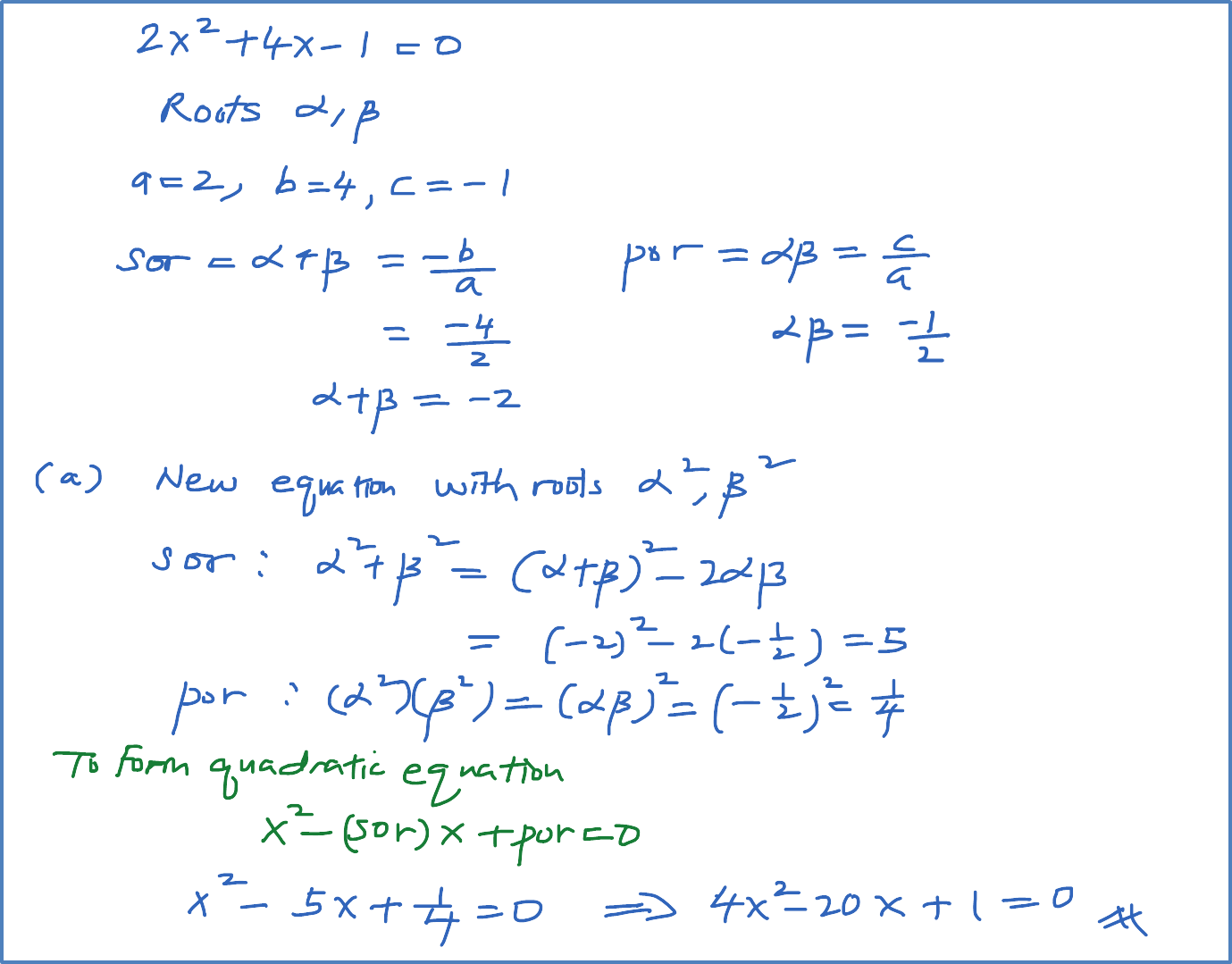
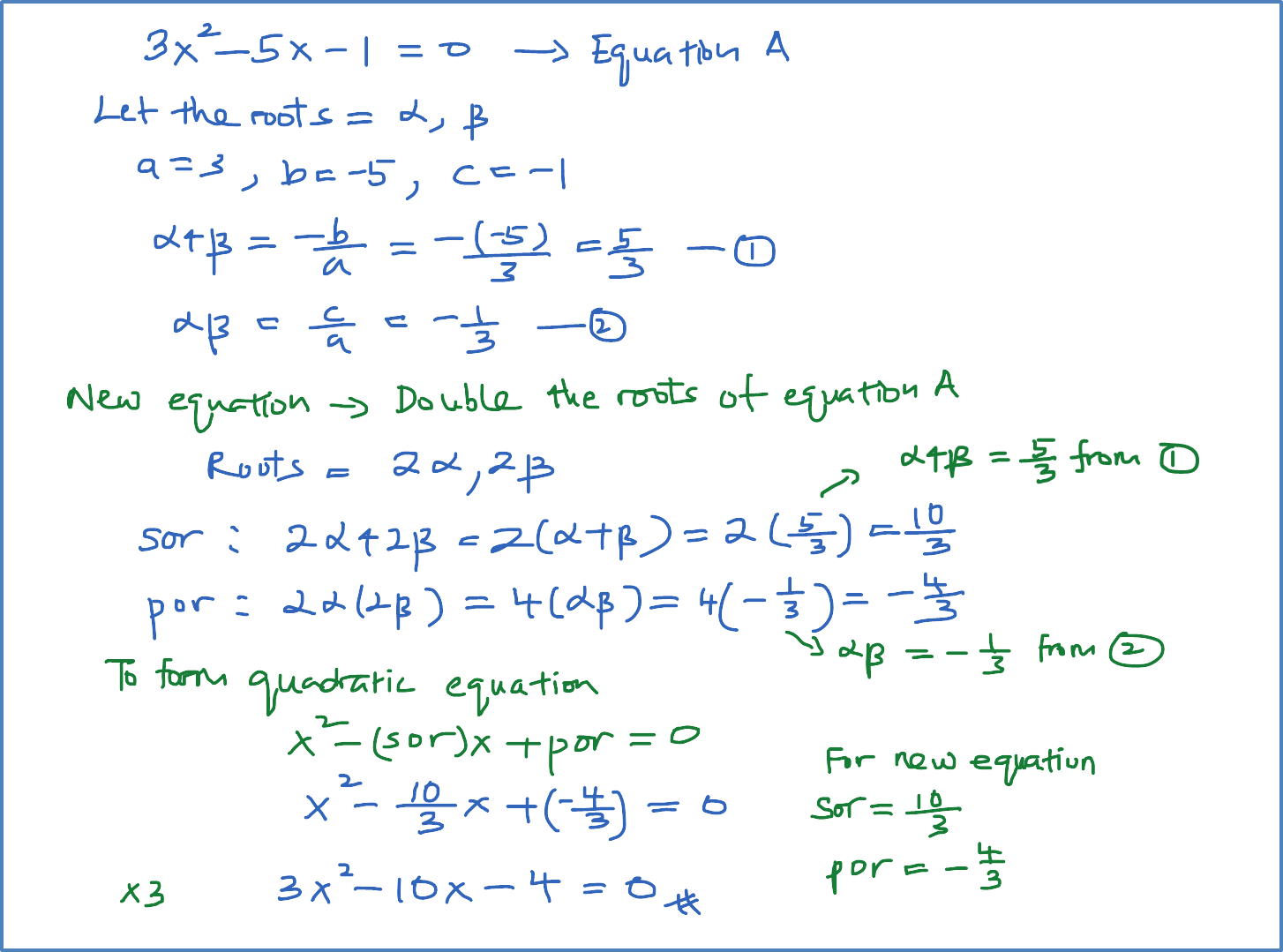
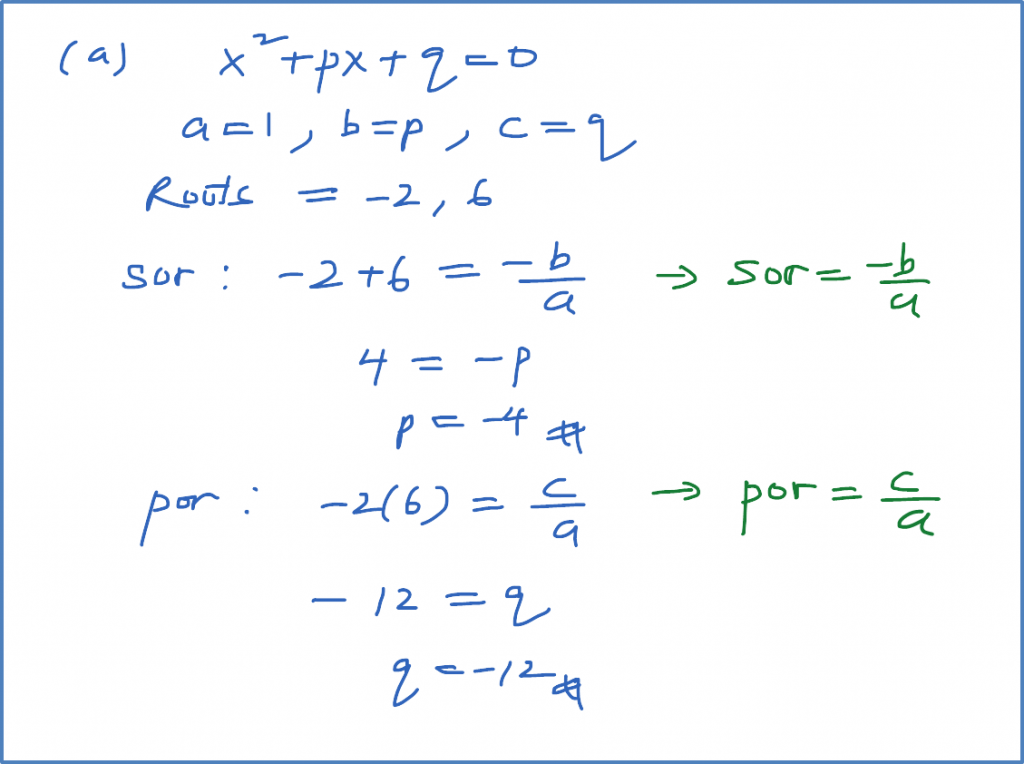Question 11:
Solve the following quadratic equation:
5x+3 x 2 1−2x =6

Solution:
5x+3 x 2 1−2x =6 5x+3 x 2 =6−12x 3 x 2 +5x+12x−6=0 3 x 2 +17x−6=0 ( 3x−1 )( x+6 )=0 3x−1=0     or     x+6=0      3x=1                   x=−6        x= 1 3                   x=−6


Question 12:
Diagram above shows a rectangle ABCD.
(a) Express the area of ABCD in terms of n.
(b) Given the area of ABCD is 60 cm2, find the length of AB.
 
Solution:
(a)
Area of ABCD
= (n + 7) × n
= (n2+ 7n) cm2

(b)
Given the area of ABCD = 60
n2+ 7n = 60
n2+ 7n – 60 = 0
(n – 5) (n + 12) = 0
n = 5 or    n = – 12 (not accepted)
 
When n = 5,
Length of AB = 5 + 7 = 12 cm



Question 13 (4 marks):
Solve the following quadratic equation:
 − 2 3x−5 = x 3x−1

Solution:
− 2 3x−5 = x 3x−1 −2( 3x−1 )=x( 3x−5 ) −6x+2=3 x 2 −5x 3 x 2 −5x+6x−2=0 3 x 2 +x−2=0 ( 3x−2 )( x+1 )=0 3x−2=0     or     x+1=0 x= 2 3      or     x=−1

SPM Practice (Paper 1)

Posted on by
Question 14:
Show that 6x−6−2k x 2 = x 2  has no real roots if k> 1 4 .

Solution:




Question 15:
The quadratic equation x 2 +px+q=0 has roots –2 and 6. Find
(a) the value of p and of q,
(b) the range of values of r for which the equation x 2 +px+q=r has no real roots.

Solution:




SPM Practice (Paper 1)

Posted on by

Question 9:
The roots of the equation 6 x 2 +hx+1=0 are α and β, whereas 3α and 3β are the roots of the equation 2 x 2 −x+k=0 . Find the value of h and k.

Solution:
6 x 2 +hx+1=0 a=6, b=h, c=1 Roots=α,β sor: α+β=− b a α+β=− h 6 .........( 1 ) por: αβ= c a αβ= 1 6 .........( 2 ) 2 x 2 −x+k=0 a=2, b=−1, c=k Roots=3α, 3β sor: 3α+3β=− b a 3( α+β )=− ( −1 ) 2 α+β= 1 6 .........( 3 ) por: 3α( 3β )= c a 9αβ= k 2 k=18αβ.........( 4 ) Substitute ( 3 ) into ( 1 ) α+β=− h 6 1 6 =− h 6 h=−1 Substitute ( 2 ) into ( 4 ) k=18αβ k=18( 1 6 ) k=3




Question 10:
Find the range of values of p for which the equation 2 x 2 +5x+3−p=0 has two real distinct roots.

Solution:

SPM Practice (Paper 1)

Posted on by

Question 3:
Solve the following quadratic equations by using quadratic formula. Give your answer in four significant figures.
(a) (x+1)(x−5)=15 (b)  x 2 +3x−2 x 2 −x−1 =3

Solution:








Question 4:
If the roots of 2 x 2 +4x−1=0 are α and β, find the equations whose roots are
(a)  α 2 , β 2 (b) α−β, β−α

Solution:







Question 5:
Write and simplify the equation whose roots are double the roots of 3 x 2 −5x−1=0 , without solving the given equation.

Solution:


Quadratic Equations, SPM Practice (Paper 2)

Posted on by

2.10.3 Quadratic Equations, SPM Practice (Paper 2)

Question 5:
Given 3t and (t – 7) are the roots of the quadratic equation 4x2 – 4x + m = 0 where m is a constant.
(a)  Find the values of t and m.
(b)  Hence, form the quadratic equation with roots 4t and 2t + 6.

Solutions:
(a)
Given 3t and (t – 7) are the roots of the quadratic equation 4x2 – 4x + m = 0
a = 4, b = – 4, c = m
Sum of roots = − b a  
3t + (t– 7) = − − 4 4  
3t + t– 7 = 1
4t = 8
t = 2

Product of roots = c a  
3t (t– 7) = m 4  
4 [3(2) (2 – 7)] = m ← (substitute t = 2)
4 [3(2) (2 – 7)] = m
4 (–30) = m
m = –120

(b)
t = 2
4t = 4(2) = 8
2t + 6 = 2(2) + 6 = 10

Sum of roots = 8 + 10 = 18
Product of roots = 8(10) = 80

Using the formula, x2– (sum of roots)x + product of roots = 0
Thus, the quadratic equation is,
x2 – 18x + 80 = 0

Quadratic Equations, SPM Practice (Paper 2)

Posted on by

Question 4:
It is given α and β are the roots of the quadratic equation x (x – 3) = 2k – 4, where k is a constant.
 (a) Find the range of values of k if α≠β. (b) Given  α 2  and  β 2  are the roots of another quadratic equation      2 x 2 +tx−4=0, where t is a constant, find the value of t and of k.

Solution:
(a) x( x−3 )=2k−4 x 2 −3x+4−2k=0 a=1, b=−3, c=4−2k                     b 2 −4ac>0 ( −3 ) 2 −4( 1 )( 4−2k )>0                9−16+8k>0                             8k>7                               k> 7 8

(b) From the equation  x 2 −3x+4−2k=0, α+β=− b a          =− −3 1          =3.............( 1 ) αβ= c a     = 4−2k 1     =4−2k.............( 2 ) From the equation 2 x 2 +tx−4=0, α 2 + β 2 =− t 2 α+β=−t.............( 3 ) α 2 × β 2 =− 4 2 αβ=−8.............( 4 ) Substitute (1)=(3), 3=−t t=−3 Substitute (2)=(4), 4−2k=−8 4+8=2k k=6

Quadratic Equations, SPM Practice (Paper 2)

Posted on by

2.10.2 Quadratic Equations, SPM Practice (Paper 2)

Question 3:
If α and β are the roots of the quadratic equation 3x2 + 2x– 5 = 0, form the quadratic equations that have the following roots.
(a)  2 α  and  2 β (b)  ( α + 2 β )  and  ( β + 2 α )

Solution:
3x2 + 2x – 5 = 0
a = 3, b = 2, c = –5
The roots are α and β.
α + β = − b a = − 2 3 α β = c a = − 5 3

(a)
The new roots are  2 α and 2 β . Sum of new roots = 2 α + 2 β = 2 β + 2 α α β = 2 ( α + β ) α β = 2 ( − 2 3 ) − 5 3 = 4 5

Product of new roots = ( 2 α ) ( 2 β ) = 4 α β = 4 − 5 3 = − 12 5

Using the formula, x2– (sum of roots)x + product of roots = 0
The new quadratic equation is
x 2 − ( 4 5 ) x + ( − 12 5 ) = 0
5x2 – 4x– 12 = 0



(b)
The new roots are  ( α + 2 β ) and ( β + 2 α ) . Sum of new roots = ( α + 2 β ) + ( β + 2 α )
= α + β + ( 2 α + 2 β ) = α + β + 2 α + 2 β α β = α + β + 2 ( α + β ) α β = 4 5 + 2 ( 4 5 ) − 12 5 = 4 5 − 2 3 = 2 15
Product of new roots = ( α + 2 β ) ( β + 2 α ) = α β + 2 + 2 + 4 α β
= − 12 5 + 4 + 4 − 12 5 = − 12 5 + 4 − 5 3 = − 1 15

The new quadratic equation is
x 2 − ( 2 15 ) x + ( − 1 15 ) = 0
15x2 – 2x– 1 = 0

Quadratic Equations, SPM Practice (Paper 2)

Posted on by


Question 2:
Given α and β are two roots of the quadratic equation (2x + 5)(x + 1) + p = 0 where αβ = 3 and p is a constant.
Find the value p, α and of β.

Solutions:
(2x + 5)(x + 1) + p = 0
2x2 + 2x + 5x + 5 + p = 0
2x2 + 7x + 5 + p = 0
*Compare with, x2– (sum of roots)x + product of roots = 0
x 2 + 7 2 x + 5 + p 2 = 0 ← divide both  sides with 2
Product of roots, αβ = 3
5 + p 2 = 3  
5 + p = 6
p = 1

Sum of roots = − 7 2  
   α + β = − 7 2   →  (1) and  α β = 3    →  (2) from (2),  β = 3 α    →  (3) Substitute (3) into (1), α + 3 α = − 7 2  

2α2+ 6 = –7α ← (multiply both sides with 2α)
2α2+ 7α + 6 = 0
(2α + 3)(α + 2) = 0
2α + 3 = 0   or α + 2 = 0
α=− 3 2    α = –2

Substitute  α = − 3 2  into (3), β = 3 − 3 2 = 3 ( − 2 3 ) = − 2

Substitute α = –2 into (3),
β = − 3 2 ∴   p = 1 ,  and when  α = − 3 2 , β = − 2  and  α = − 2 , β = − 3 2 .


Quadratic Equations, SPM Practice (Paper 2)

Posted on by

2.10.1 Quadratic Equations, SPM Practice (Paper 2)

Question 1:
(a)  Find the values of k if the equation (1 – k) x2– 2(k + 5)x + k + 4 = 0 has real and equal roots.
Hence, find the roots of the equation based on the values of k obtained.
(b)  Given the curve y = 5 + 4x – x2 has tangen equation in the form y = px + 9. Calculate the possible values of p.

Solutions:
(a)
For equal roots,
b2 – 4ac = 0
[–2(k + 5)] 2 – 4(1 – k)( k + 4) = 0
4(k + 5) 2 – 4(1 – k)( k + 4) = 0
4(k2 + 10k + 25) – 4(4 – 3k – k2) = 0
4k2 + 40k + 100 – 16 + 12k + 4k2 = 0
8k2 + 52k + 84 = 0
2k2 + 13k + 21 = 0
(2k + 7) (k + 3) = 0
k = − 7 2 ,   − 3

If k = − 7 2  , the equation is
( 1 + 7 2 ) x 2 − 2 ( − 7 2 + 5 ) x − 7 2 + 4 = 0 9 2 x 2 − 3 x + 1 2 = 0  

9x2 – 6x + 1 = 0
(3x – 1) (3x – 1) = 0
x = ⅓

If k = –3, the equation is
(1 + 3)x 2 – 2(–3 + 5)x – 3 + 4 = 0
4x2 – 4x + 1 = 0
(2x – 1) (2x – 1) = 0
x = ½

(b)
y = 5 + 4x – x2 ----- (1)
y = px + 9 ---------- (2)
(1)  = (2), 5 + 4x – x2= px + 9
x2 + px – 4x + 9 – 5 = 0
x2 + (p – 4)x + 4 = 0

Tangen equation only has one intersection point with equal roots.
b2 – 4ac = 0
(p – 4)2 – 4(1)(4) = 0
p2 – 8p + 16 – 16 = 0
p2 – 8p = 0
p (p – 8) = 0
Therefore, p = 0 and p = 8.

2.2a Solving Quadratic Equations – Factorisation

Posted on by
2.4.1 Solving Quadratic Equations – Factorisation
1. If a quadratic equation can be factorised into a product of two factors such that

(x – p)(x – q) = 0

Hence
 x – p = 0   or  x – q = 0
   x = p   or x = q

p and q  are the roots of the equation.

Notes
1.The equation must be written in general form ax2 + bx+ c = 0 before factorisation.
2. This method can only be used if the quadratic expression can be factorised completely.



Example 1:
Find the roots of the quadratic equations
(a) x (2x − 8) = 0 
(b) x2 −16x = 0
(c) 3x2 − 75x = 0
(d) 5x2 − 100x = 25x

Solution:
(a) 
x (2x − 8) = 0 
x = 0  or  2x − 8 = 0
2x − 8 = 0
2x = 8
x= 4
x = 0  or  x = 4

(b)
x2 −16x = 0
x (x − 16) = 0 
x = 0  or  x − 16 = 0
x = 0  or  x = 16

(c) 
3x2 − 75x = 0
3x (x − 25) = 0 
3x = 0  or  x − 25 = 0
x = 0  or  x = 25

(d) 
5x2 − 100x = 25x
5x2 − 100x − 25x = 0
5x2 − 125x = 0
x (5x − 125) = 0 
x = 0  or  5x − 125 = 0
5x = 125
x = 25
x = 0  or  x = 25



Example 2:
Solve the following quadratic equations
(a) x2 − 4x – 5 = 0
(b) 1 − 5x + 2x2 = 4

Solution:
(a) 
x2 − 4x – 5 = 0
(x – 5) (x + 1) = 0
x – 5 = 0  or  x + 1 = 0
x = 5  or  x = –1

(b)
1 − 5x + 2x2 = 4
2x2 − 5x + 1 – 4 = 0
2x2 − 5x – 3 = 0
(2x + 1) (x – 3) = 0
2x + 1= 0  or  x – 3 = 0
2x = –1  or  x = 3
x = –½  or  x = 3