Question 14 (4 marks):
An aquarium has the length of (x + 7) cm, the width of x cm and the height of 60 cm.
The total volume of the aquarium is 48000 cm3. The aquarium will be filled fully with water.
Calculate the value of x.
Solution:
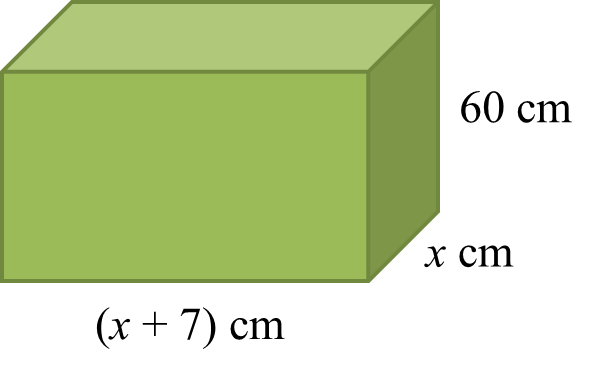
An aquarium has the length of (x + 7) cm, the width of x cm and the height of 60 cm.
The total volume of the aquarium is 48000 cm3. The aquarium will be filled fully with water.
Calculate the value of x.
Solution:

Question 15 (4 marks):
Diagram 3 shows a garden path with a rectangular shape. There are 8 similar circular stepping stone built in the path.

Given the area of the path is 32 m2, find the diameter, in m, of one piece of stepping stone.
Solution:
Diagram 3 shows a garden path with a rectangular shape. There are 8 similar circular stepping stone built in the path.

Given the area of the path is 32 m2, find the diameter, in m, of one piece of stepping stone.
Solution:
2.3 Quadratic Equations
2.3 Quadratic Equations
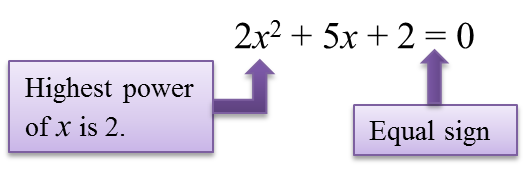
1. Quadratic equations are equations which fulfil the following characteristics:
(a) Have an equal ‘=’ sign
(b) Contain only one unknown
(c) Highest power of the unknown is 2.
For example,

2. The general form of a quadratic equation is written as:
(a)
ax2 + bx + c = 0,
ax2 + bx + c = 0,
where a ≠ 0, b ≠ 0 and c ≠ 0,
example: 4x2+ 13x – 12 = 0
(b)
ax2 + bx = 0,
where a ≠ 0, b ≠ 0 but c = 0,
example: 7x2+ 9x = 0
(c)
ax2 + c = 0,
where a ≠ 0, c ≠ 0 but b = 0,
example: 9x2– 3 = 0
Example 1:
(b) –2 + 5x2– 6x = 0
(c) 7p2 – 3p = 4p2+ 4p – 3
(d) (x – 2)(x + 6) = 0
(e) 3 – 13x = 4 (x2 + 2)
(f)
(g)
(h)
(i)
Write each quadratic equation in the general form.
(a) x2 – 5x = 12
(b) -2 + 5x2– 6x = 0
(c) 7p2 – 3p = 4p2+ 4p – 3
(d) (x – 2)(x + 6) = 0
(e) 3 – 13x = 4 (x2 + 2)
(f)
(g)
(h)
(i)
Solution:
(g)
(h)
(i)
Solution:
A quadratic equation in the general form is written as ax2 + bx + c = 0
(a) x2 – 5x = 12
x2 – 5x -12 = 0
(b) –2 + 5x2– 6x = 0
5x2 – 6x –2 = 0
(c) 7p2 – 3p = 4p2+ 4p – 3
7p2 – 3p – 4p2– 4p + 3 = 0
3p2 – 7p + 3 = 0
(d) (x – 2)(x + 6) = 0
x2 + 6x – 2x– 12 = 0
x2 + 4x – 12 = 0
(e) 3 – 13x = 4 (x2 + 2)
3 – 13x = 4x2 + 8
–4x2 – 8 + 3 – 13x = 0
–4x2 – 13x – 5 = 0
4x2 + 13x + 5 = 0
(f)
2y – y2 = 1 – 3y
2y – y2 – 1 + 3y = 0
– y2 + 5y – 1 = 0
y2 – 5y + 1 = 0
(g)
10p = 8p2 – 12
–8p2 + 10p +12 = 0
8p2 – 10p – 12 = 0
(h)
2y2 + 10 = 4y – 4
2y2 – 4y + 10 + 4 = 0
2y2 – 4y + 14 = 0
2y2 – 4y + 14 = 0
(i)
4p = 7p (7p– 6)
4p = 49p2 – 42p
– 49p2 + 42p + 4p = 0
49p2 – 46p = 0
Quadratic Equations Long Questions (Question 5 – 7)
Question 5:
Solution:
Solve the equation:
(m + 2)(m – 4) = 7(m – 4).
(m + 2)(m – 4) = 7(m – 4)
m2– 4m + 2m – 8 = 7m – 28
m2– 9m + 20 = 0
(m – 5)(m – 4) = 0
m = 5 or m = 4
Question 6:
Solution:
Solve the equation:
Solution:
Question 7:
Solution:
Solve the equation:
Solution:
2.4 Roots of Quadratic Equations
2.4 Roots of Quadratic Equations
1. A root of quadratic equation is the value of the unknown which satisfies the quadratic equation.
2. Roots of an equation are also called the solution of an equation.
2. Roots of an equation are also called the solution of an equation.
3. To solve a quadratic equation by the factorisation method, follow the steps below:
Step 1: Express the quadratic equation in general form ax2 + bx + c = 0.
Step 2: Factorise the quadratic expression ax2 + bx + c = 0 as the product of two linear expressions, that is, (mx+ p) (nx + q) = 0.
Step 3: Equate each factor to zero and obtain the roots or solutions of the quadratic equation.
Example 1:
Solution:
Example 2:
Example 3:
Solution:
Solve the quadratic equation
Solution:
2x2 – 5 = 9x
2x2 – 9x – 5 = 0
(x – 5)(2x + 1) = 0
x – 5 = 0, x = 5
or 2x + 1 = 0
Therefore, x = 5 and x = ½ are roots or solutions of the quadratic equation.
Example 2:
Solve the quadratic equation 4x2 – 12 = –13x
Solution:
4x2 – 12 = –13x
4x2 + 13x – 12 = 0
(4x – 3)(x + 4) = 0
4x – 3 = 0,
or x + 4 = 0
x = –4
Example 3:
Solve the quadratic equation 5x2 = 3 (x + 2) – 4
Solution:
5x2 = 3 (x + 2) – 4
5x2 = 3x + 6 – 4
5x2 – 3x – 2 = 0
(5x + 2)(x – 1) = 0
5x + 2 = 0,
or x – 1 = 0
x = 1
Example 4:
Example 4:
Solve the quadratic equation
Solution:
3x2 – 9x = – 4x + 12
3x2 – 5x – 12 = 0
(3x + 4)(x – 3) = 0
3x + 4 = 0,
or x – 3 = 0
x = 3
2.1 Quadratic Expression (Sample Questions)
Example 1:
Solution:
(a) (6p – 2)(2p – 1)
(b) (m + 5)(4 – 7m)
(c) (x + 2) (2x – 3)
Form a quadratic expression by multiplying each of the following.
(a) (6p – 2)(2p – 1)
(b) (m + 5)(4 – 7m)
(c) (x + 2) (2x – 3)
Solution:
= (6p)(2p) + (6p)(-1) + (-2)(2p) +(-2)(-1)
= 12p2 – 6p – 4p + 2
= 12p2 – 10p + 2
(b) (m + 5)(4 – 7m)
= (m)(4) + (m)(-7m) + (5)(4) + (5)(-7m)
= 4m – 7m2 + 20 – 35m
= – 7m2 – 31m+ 20
(c) (x + 2) (2x – 3)
= (x)(2x) + (x)(-3) + (2)(2x) + (2)(-3)
= 2x2-3x + 4x – 6
= 2x2 + x - 6
Quadratic Equations Long Questions (Question 8 – 10)
Question 8:
Solve the following quadratic equation:
4x (x + 4) = 9 + 16x
Solution:
Solve the following quadratic equation:
4x (x + 4) = 9 + 16x
Solution:
Question 9:
Solve the following quadratic equation:
(x + 2)2 = 2x + 7
Solution:
Solve the following quadratic equation:
(x + 2)2 = 2x + 7
Solution:
Question 10:
Solve the following quadratic equation:
Solution:
Solve the following quadratic equation:
Solution:
2.2 Factorisation of Quadratic Expression
2.2 Factorisation of Quadratic Expression
(A) Factorisation quadratic expressions of the form ax2 + bx + c, b = 0 or c = 0
1. Factorisation of quadratic expressions is a process of finding two linear expressions whose product is the same as the quadratic expression.
2. Quadratic expressions ax2 + c and ax2 + bx that consist of two terms can be factorised by finding the common factors for both terms.
Example 1:
Factorise each of the following:
(a) 2x2+ 6
(b) 7p2– 3p
(c) 6x2– 9x
Solution:
(a) 2x2+ 6 = 2 (x2 + 3) ← (2 is common factor)
(b) 7p2– 3p = p (7p – 3) ← (p is common factor)
(c) 6x2– 9x = 3x (2x – 3) ← (3x is common factor)
(B) Factorisation of quadratic expressions in the form ax2 – c , where a and c are perfect squares
Example 2:
(a) 9p2– 16
(b) 25x2– 1
(c)
Solution:
(a) 9p2– 16 = (3p)2 – 42= (3p – 4) (3p + 4)
(b) 25x2– 1 = (5x)2 – 12= (5x – 1) (5x + 1)
(c)
(C) Factorisation quadratic expressions in the form ax2 + bx + c, where a ≠ 0, b ≠ 0 and c ≠ 0
Example 3:
Factorise each of the following
(a) 3y2+ 2y – 8
(b) 4x2– 12x + 9
Solution:
(a)
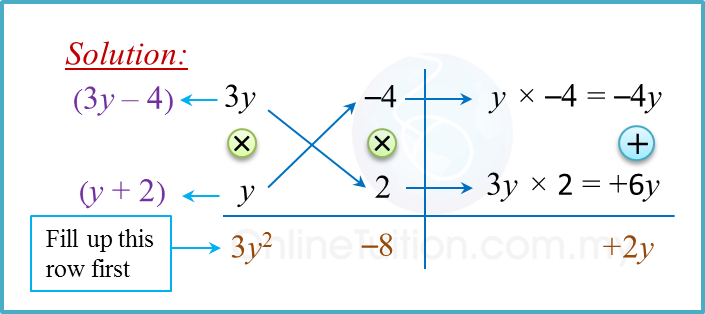
Factorise using the Cross Method

3y2+ 2y – 8 = (3y – 4) (y + 2)
(b)

(b)

4x2– 12x + 9 = (2x – 3) (2x – 3)
2.1 Quadratic Expression
(A) Identifying quadratic expression
1. A quadratic expression is an algebraic expression of the form ax2 + bx + c, where a, b and c are constants, a ≠ 0 and x is an unknown.
(a) The highest power of x is 2.
(b) For example, 5x2– 6x + 3 is a quadratic expression.
Example 1
State whether each of the following is a quadratic expression in one unknown.
(a) x2 – 5x + 3
(b) 8p2 + 10
(c) 5x + 6
(d) 2x2 + 4y + 14
(e)
(f) y3 – 3y + 1
Solution:
(a) Yes. A quadratic expression in one unknown.
(b) Yes. A quadratic expression in one unknown.
(c) Not a quadratic expression in one unknown. The highest power of the unknown x is not 2.
(d) Not a quadratic expression in one unknown. There are 2 unknowns, x and y in the quadratic expression.
(e) Not a quadratic expression in one unknown. The highest power of the unknown x is not 2.
(f) Not a quadratic expression in one unknown. The highest power of the unknown x is not 2.
2. A quadratic expression can be formed by multiplying two linear expressions.
(2x + 3)(x - 3) = 2x2 – 3x – 9
Example 2
Multiply the following pairs of linear expressions.
(a) (4x + 3)(x – 2)
(b) (y – 6)2
(c) 2x (x – 5)
Solution:
(a) (4x + 3)(x – 2)
= (4x)(x) + (4x)(-2) +(3)(x) + (3)(-2)
= 4x2 – 8x + 3x – 6
= 4x2 – 5x – 6
(b) (y – 6)2
= (y – 6)(y – 6)
= (y)(y) + (y)(-6) + (-6)(y) + (-6)(-6)
= y2 -6y – 6y + 36
= y2 - 12y + 36
(c) 2x (x – 5)
= 2x(x) + 2x(-5)
= 2x2 – 10x
Quadratic Equations Long Questions (Question 1 – 4)
Question 1:
Solution:
Solve the quadratic equation, (y + 3)(y – 4) = 30
Solution:
(y + 3)(y – 4) = 30
y2 – 4y + 3y– 12 = 30
y2 – y – 12 – 30 = 0
y2 – y – 42 = 0
(y + 6)(y – 7) = 0
y + 6 = 0, y = –6
Or
y – 7 = 0
y = 7
Question 2:
Solution:
Solve the quadratic equation, 5x2 = 3( x – 2) + 8
Solution:
5x2 = 3x – 6 + 8
5x2 – 3x – 2 = 0
(5x + 2)(x – 1) = 0
5x + 2 = 0, x
Or
x – 1 = 0
x = 1
Question 3:
Solution:
Solve the quadratic equation
Solution:
2p2 – 15 = 7p
2p2 –7p – 15 = 0
(2p + 3)(p – 5) = 0
2p + 3 = 0, p =
Or
p – 5 = 0
p = 5
Question 4:
Solution:
( ×2), 2y2 – 9y= 5
Solve the quadratic equation
Solution:
( ×2), 2y2 – 9y= 5
2y2 – 9y – 5 = 0
(2y + 1)(y – 5) = 0
2y + 1 = 0, y = – ½
Or
y – 5 = 0
y = 5